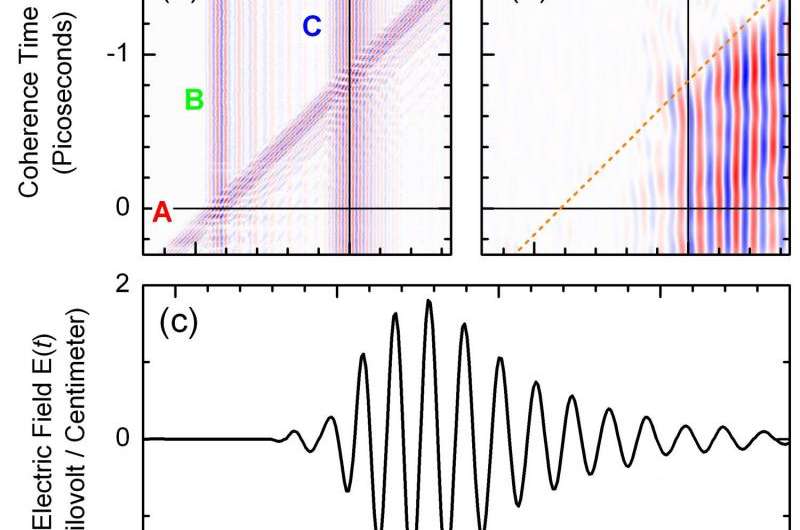
Fig. 1: Experimental data: (a) Two-dimensional (2D) scan of the sum of the electric fields E(τ,t) of the three driving THz pulses A, B, and C as a function of the coherence time τ and the real time t. The contour plot is colored red for positive electric fields and blue for negative fields. (b) 2D scan of electric field ENL(τ,t) nonlinearly emitted by the two-phonon coherence in InSb. The orange dashed line indicates the center of pulse A. (c) Electric field transient ENL(0,t) for the coherence time τ=0.
Two-quantum oscillations of atoms in a semiconductor crystal are excited by ultrashort terahertz pulses. The terahertz waves radiated from the moving atoms are analyzed by a novel time-resolving method and demonstrate the non-classical character of large-amplitude atomic motions.
The classical pendulum of a clock swings forth and back with a well-defined elongation and velocity at any instant in time. During this motion, the total energy is constant and depends on the initial elongation which can be chosen arbitrarily. Oscillators in the quantum world of atoms and molecules behave quite differently: their energy has discrete values corresponding to different quantum states. The location of the atom in a single quantum state of the oscillator is described by a time-independent wavefunction, meaning that there are no oscillations.
Oscillations in the quantum world require a superposition of different quantum states, a so-called coherence or wavepacket. The superposition of two quantum states, a one-phonon coherence, results in an atomic motion close to the classical pendulum. Much more interesting are two-phonon coherences, a genuinely non-classical excitation for which the atom is at two different positions simultaneously. Its velocity is nonclassical, meaning that the atom moves at the same time both to the right and to the left as shown in the movie. Such motions exist for very short times only as the well-defined superposition of quantum states decays by so-called decoherence within a few picoseconds (1 picosecond = 10-12 s). Two-phonon coherences are highly relevant in the new research area of quantum phononics where tailored atomic motions such as squeezed and/or entangled phonons are investigated.
In a recent issue of Physical Review Letters, researchers from the Max Born Institute in Berlin apply a novel method of two-dimensional terahertz (2D-THz) spectroscopy for generating and analyzing non-classical two-phonon coherences with huge spatial amplitudes. In their experiments, a sequence of three phase-locked THz pulses interacts with a 70-μm thick crystal of the semiconductor InSb and the electric field radiated by the moving atoms serves as a probe for mapping the phonons in real-time. Two-dimensional scans in which the time delay between the three THz pulses is varied, display strong two-phonon signals and reveal their temporal signature [Fig. 1]. A detailed theoretical analysis shows that multiple nonlinear interactions of all three THz pulses with the InSb crystal generate strong two-phonon excitations.
This novel experimental scheme allows for the first time to kick off and detect large amplitude two-quantum coherences of lattice vibrations in a crystal. All experimental observations are in excellent agreement with theoretical calculations. This new type of 2D THz spectroscopy paves the way towards generating, analyzing, and manipulating other low-energy excitations in solids such as magnons and transitions between ground and excited states of excitons and impurities with multiple-pulse sequences.
Visualization of nonclassical quantum coherences in matter. The two parabolas (black curves) show the potential energy surfaces of harmonic oscillators representing the oscillations of atoms in a crystalline solid around their equilibrium positions, i.e., the so called phonons. Blue curves: probability of presence of atoms at different spatial positions in thermal equilibrium. The quantum mechanical uncertainty principle demands a finite width of such distribution functions. Red curves: time-dependent probability distributions of coherent oscillating states in matter. One-phonon coherence (left panel): the quantum mechanical motion of atoms resembles the classical motion of a pendulum (cyan ball). The latter moves during the oscillation either from left to right or vice versa. Two-phonon coherence (right panel): quantum mechanics allows also for kicking off a nonclassical state with the quantum-mechanical property that the atom can be at two positions simultaneously. The velocity of the atoms behaves also nonclassical, i.e., the atom moves at the same time both to the right and to the left. In the case of a perfect harmonic oscillator the currents of the two parts of the atom exactly cancel each other. Thus, a small anharmonicity is necessary to observe the emission of a coherent electric field transient as shown in Fig. 1(c).
More information: Carmine Somma et al. Two-Phonon Quantum Coherences in Indium Antimonide Studied by Nonlinear Two-Dimensional Terahertz Spectroscopy, Physical Review Letters (2016). DOI: 10.1103/PhysRevLett.116.177401
Journal information: Physical Review Letters
Provided by Forschungsverbund Berlin e.V. (FVB)