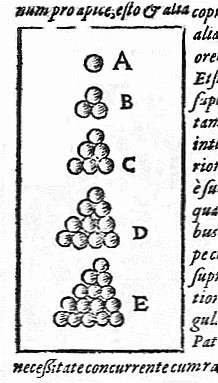
One of the diagrams from Strena Seu de Nive Sexangula, illustrating the Kepler conjecture
(Phys.org) —A team of researchers led by the man, Thomas Hales, who came up with written proof of the Kepler conjecture is now reporting that they have constructed a formal proof of the conjecture, which implies the use of a computer. The announcement was made on The Flyspeck Project page, and puts to rest any doubts about the correctness of the proof done by Hales in 1998.
It was back in 1611 that Johannes Kepler first suggested in a paper he'd written (after corresponding with Thomas Harriot) that if you want to stack cannon balls on a ship's deck in the most efficient way possible (a question originally posed by Sir Walter Raleigh), the best way to do it is in a pyramid shape. Cut to 1998 when Hales completed work on his written proof (which included portions that could only be verified by computer). But it was huge, as many mathematical proofs tend to be—300 pages in all that took 12 reviewers four years to verify. A 99 percent certainty rating wasn't enough for Hales, however, so he launched the Flyspeck Project to create a means for proving the proof correct using a computer.
Hales and his team used two software tools that have been created for the sole purpose of verifying proofs—HOL Light and Isabelle—they work by checking the logic of statements made in the proof. Now after eleven years of work, the team has announced that they've succeeded in their endeavor. Hales' original proof has been converted to code the computer can work with and the software checkers found no discrepancies, which means the proof of the conjecture has been verified.
The success by the team highlights a growing movement of using computers to verify proofs that human beings have created giving the results more credence. Computers have also been used to construct original proofs for some basic problems, which suggests that someday in the not-too-distant future, computers will be doing all the heavy work, leaving mathematicians to ponder the deeper questions regarding what the proofs represent and how they can be used in real world applications.
More information: code.google.com/p/flyspeck/wik … AnnouncingCompletion
via Newscientist
© 2014 Phys.org