Load-bearing entanglements in polymer glasses

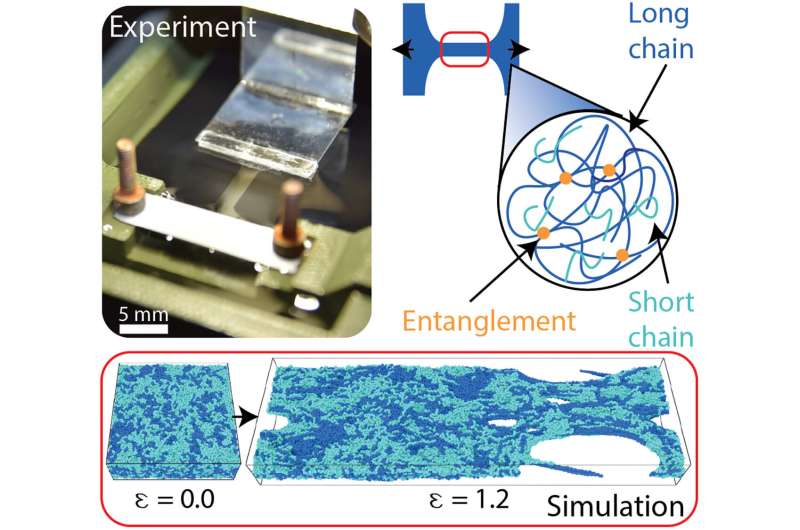
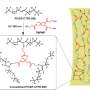
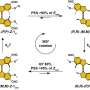
The role of entanglements can determine the mechanical properties of glass polymer blends. In a new report now published on Science Advances, Cynthia Bukowski and a research team in polymer science and biomolecular engineering at the University of Massachusetts and the University of Pennsylvania, U.S., developed a combined method of experiments and simulations to quantify the role of entanglements on polymer glasses. The team conducted uniaxial extension experiments on 100 nm thin films with a bidispersive mixture of polystyrene, for comparison with molecular dynamics simulations of a coarse-grained model of polymer glasses. The bidispersive blends allowed systematic tuning of the entanglement density present in both systems and the scientists measured the film strength experimentally and described the simulated film toughness using a model to account for load-bearing entanglements.

Breaking glassy polymers

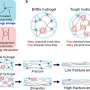
Glassy polymers are important for a range of technologies from additive manufacturing to clean water filtration membranes. The stiffness and processability of the materials make them attractive for many applications for researchers to determine their lifetime and performance limits. The entanglements between polymer molecules in the glassy state play a significant role to determine strength. Glassy polymer materials are excessively brittle below a critical number of entanglements per molecule and can break at diminishing levels of stress. Polymer materials can dissipate energy above a critical entanglement density to enhance their strength and toughness. These processes are unique and give rise to wide-ranging technologies. Enlargements are important for strength but require volatile and costly solvents or excessive temperatures during processing. In this work, Bukowski et al. combined new experimental strategies with molecular dynamics simulations to reveal how all entanglements did not equally contribute to a polymer glass. The team developed and validated a scaling theory to describe the number of strengthening entanglements per polymer chain to provide a framework to maximize strength with minimal entanglements in a polymer glass.

The interplay of forces

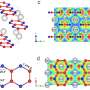
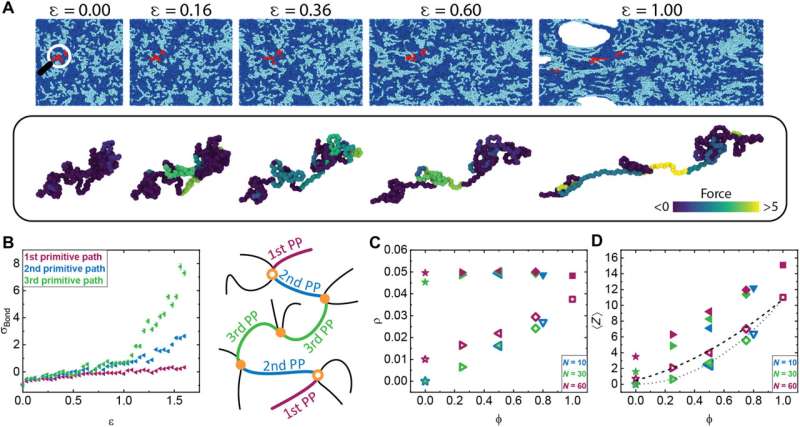
The mechanical properties of the polymer glasses depended on the interplay of van der Waals forces and entanglements. Plastic deformation is often associated with shear deformation zones that precede crack growth. Entanglements play a significant role in shear deformation zones and their stability or resistance to form a crack is a function of entanglement density as well as temperature and strain rate. These deformations can be tracked experimentally with optical and transmission electron microscopy. Bukowski et al. overcame existing limits of the study by using a recently developed experimental method to measure the stress-strain response of ultrathin polymer films. Molecular dynamics (MD) simulations have provided valuable insights to the process of entanglements in polymer melts and glasses, where the simulations agreed very well with the experiments. In addition to the simulations, the scaling theories also contributed to define the role of entanglements in the mechanical properties of polymer glasses. The combined approach of experimental and MD approaches allowed the team to examine the macroscopic perspective of experimental films and the molecular view of local dynamics simulations, to understand polymer strength.

Mechanical properties of polymer blends and counting entanglements

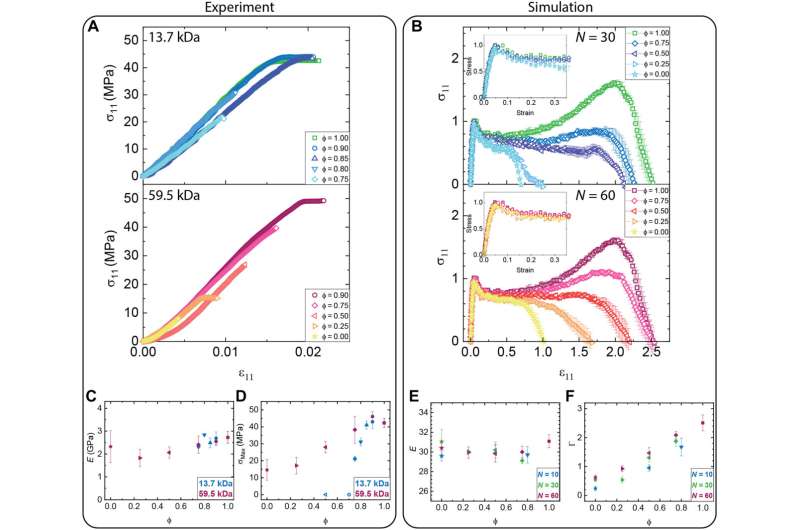
Bukowski et al. regulated the number of entanglements in the system by mixing monodisperse polymers of the same chemical structure. This method allowed them to sample a wide range of entanglements per chain. Both experiments and simulations provided qualitative insights to understand how the entanglement network contributed to the mechanical properties of glassy polymer materials. During the experiments, when the concentration of the longest chains decreased, the maximum stress and failure strain also began to decrease. The team noted different failure mechanisms by varying the degree of dilution and dictated the elastic modulus of the glassy state of the polymer by local intersegment interactions dominated by van der Waals forces. As Bukowski et al. integrated long chain polymers, the toughness measured in the simulations revealed a monotonic increase.

Experiments and simulations

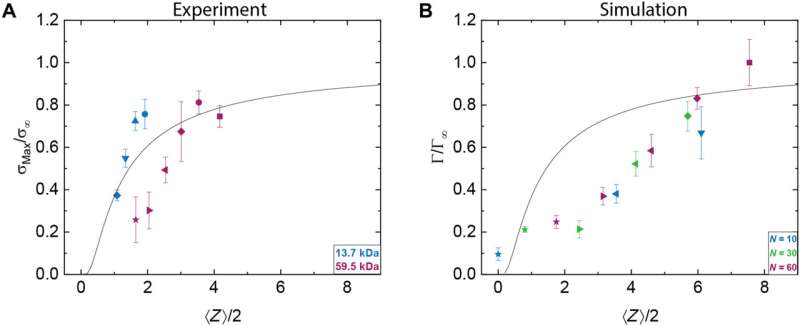
The experimental maximum stress and simulated toughness values appeared to scale differently for different experimental models. The findings matched well with a physics model introduced by Mikos and Peppas. According to the simulations, not all entanglements in a system were load bearing. Later, the team noted the simulated strains to be much larger than those observed experimentally. To precisely account for changes in load-bearing entanglements, the team considered the average number of entanglements per chain. Bukowski et al. next developed a model and successfully applied the method to both experiments and simulations to study the relationship between entanglements and the maximum stress or toughness. When considering the load-bearing fraction of entanglements in the system, the experiments and simulations scaled similarly at the molecular level. By comparing the experiment and simulations of thin glassy polymer films thereafter, the data indicated a quantitative link between the maximum stress and toughness.

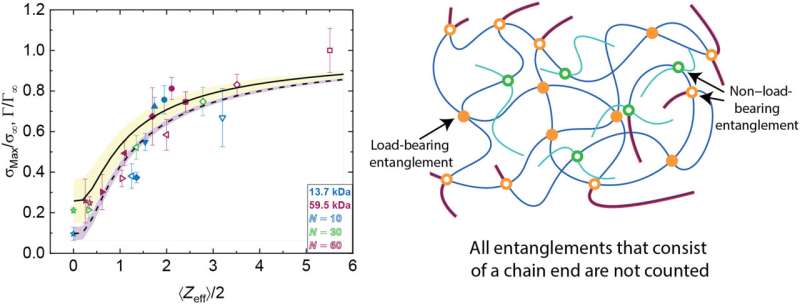
Outlook

In this way, Cynthia Bukowski and colleagues used a combination of experiments and simulations to show the importance of using load-bearing entanglements to improve the toughness of materials. To accomplish this, they systematically tuned the entanglement density using bidisperse and chemically identical blends. When they added a short chain diluent to the blends, the tensile tests of the polymer showed a decreased maximum stress. Similarly, the molecular dynamics of solutions also showed a decrease in the toughness with added diluent. The combined experiments and simulations provided an enhanced understanding of polymers and a novel framework to tune the mechanical properties based on their molecular makeup. The work has many practical implications beyond providing fundamental insights into the origin and toughness in polymer glasses, including additive manufacturing strategies.

© 2021 Science X Network