Study unveils strain-induced quantum phase transitions in magic-angle graphene

Over the past few years, many physicists and material scientists worldwide have been investigating the properties and characteristics of magic-angle twisted bilayer graphene (MATBG). MATBG is a strongly correlated material that was first experimentally realized in 2018. This unique material hosts a diverse array of highly correlated phases, including metals, semimetals, Chern insulators, quantum anomalous hall states and, perhaps most interestingly, superconductivity.

Researchers at University of California, Berkeley (UC Berkeley) have recently carried out a study investigating the effects of uniaxial heterostrain on the interacting phase diagram of MATBH. Their findings, published in Physical Review Letters, suggest that small strain values led to a zero-temperature phase transition between two states, namely the symmetry-broken Kramers intervalley-coherent insulator and nematic semimetal phases.

"A key goal of our field is to understand the origin of superconductivity in MATBG and flesh out the mechanism responsible," Daniel Parker and Tomo Soejima, two of the researchers who carried out the study, told Phys.org via email. "However, there is an important puzzle of the MATBG phase diagram, which complicates any attempt to divine the nature of the superconductivity, namely at charge neutrality, some experiments find a semimetallic state, while other see insulators. Our work proposes that a particular phase transition may resolve this discrepancy."

All actions and changes in MATBG occur in what are known as its active bands. These bands include 2 Chern bands, times 2 valleys and times 2 spins, for a total of 8. Scientists can easily adjust the number of electrons in the system experimentally, which in turn allows them to tune these bands from all empty to all full.

"As an analogy, one can think of this like having 8 buckets that can be filled with water," Parker explained. "For a given amount of water, the MATBG picks out one, and only one, way to distribute the water. For instance, if there are two buckets worth of water, then MATBG might choose to fill 2 buckets full to the brim, or to fill 4 buckets each halfway. The phase of the system is labeled by two things: 1. how the water (electrons) is distributed in the buckets (bands) and 2. how hard is it to add one more drop of water (i.e., whether the system is insulating or conducting)."

While the insulating or conducting nature of a system is fairly easy to infer experimentally, the distribution of electrons in the bands of MATBH much harder to determine. In their paper, Parker, Soejima and their colleagues wanted to explore what happens when the number of electrons is such that it cancels the charge of carbon atoms (known as the charge neutrality point) or, when considering the water buckets analogy, if buckets are halfway filled with water.

While some past studies investigating this have observed insulating states (i.e., where it is hard to add "one more drop"), others have observed metals or semimetallic states instead. From a theoretical standpoint, previous work by Nick Bultinck and his collaborators suggests that the insulating state could be a Kramers-intervalley coherent (KIVC) state. To explain this using the water bucket analogy, it would be as if all buckets were filled halfway, but they were strangely paired up with one partner filled only on the left half and the other filled only on the right.

"Further work by Bultinck and his colleagues showed that this state is one possible origin for superconductivity in MATBG," Parker and Soejima said. "The alternative semimetallic phase is much more conventional, where the bottom half of each bucket is filled. The primary question we sought to answer was why, when previous theory predicted a KIVC state, one might observe the semimetal instead."

A possible reason for the discrepancies in past observations is that different devices have slightly different Hamiltonians. Some teams were able to use a simplified model of MATBG, first introduced by Bistrizter and McDonald, to investigate the properties of MATBG samples.

Recent studies, however, revealed that in its original form, the so-called BM model, does not capture non-local tunneling present in DFT, alignment with hBN substrate, and renormalization of free-fermion bandstructure, and other effects. Parker, Soejima and their colleagues thus wanted to determine what effect could be considered to explain the observed discrepancy.

"Bultinck had a shrewd suspicion that strain might be the culprit responsible for this discrepancy," Parker and Soejima said. "While a realistic way to model strain in MATBG had already been proposed and its effect on non-interacting band structure (i.e., solution of the Hamiltonian without Coulomb interaction) had been investigated, its effect on the phase diagram in the presence of interaction had not been investigated so far."

To test the hypothesis introduced by Bultinck, the researchers used two complementary numerical techniques, known as self-consistent Hartree-Fock (HF) and density-matrix renormalization group (DMRG). Hartree-Fock is a standard approximation that incorporates the most important effects of electron-electron interactions. This approximation is highly flexible; thus, it allows researchers to examine large system sizes of 24 x 24-unit cells.

"Since HF is an approximation, there is always the scary possibility that it is producing a 'false' phase," Parker and Soejima said "We thus used DMRG to rule this out. DMRG is an unbiased numerical technique which, with sufficient computational power, will determine the true phase of the system. Using it for 2D systems with long-range interactions as we have here is non-trivial, and requires special techniques developed by us in an earlier paper."

Compared to HF approximation, DMRG is slower, more expensive and can only be used to examine small systems. To achieve reliable results, Parker, Soejima and their colleagues thus decided to use HF and DMRG in tandem, as HF allowed them to map out the entire phase diagram and DMRG to verify that the HF approximation was correct.

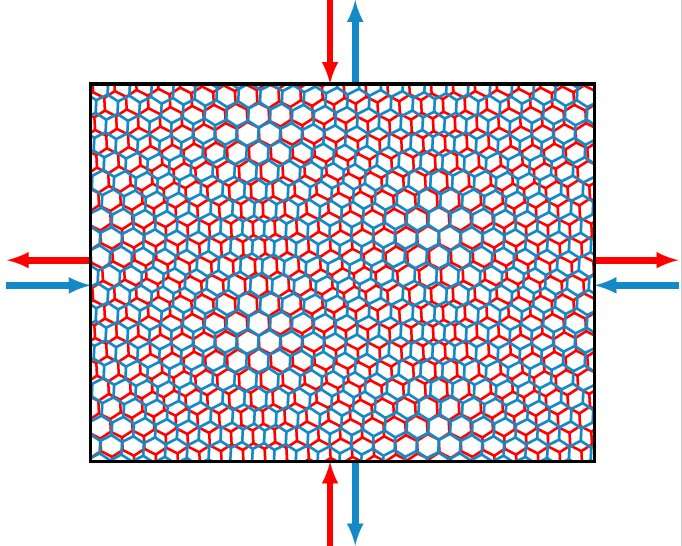
"The key finding of our work is that small amounts of heterostrain (precisely in the ε∼0.1%–0.2% range) can destroy the KIVC phase and replace it with a semimetal," Parker and Soejima said. "Any sheet of graphene made in the lab is always under some stress, which compresses it in one direction while stretching it in the other. In MATBG, one has the additional possibility of heterostrain, where the top layer is compressed along stretching axis of the bottom layer, and vice versa."

In the past, some researchers carried out experiments measuring the heterostrain present in MATBG samples and found that it was tiny, ranging between 0.1% - 0.7%. When Parker, Soejima, and their colleagues first started exploring this topic, they were fairly skeptical that such a small amount of strain would have particular effects, thus their results came as a surprise to them.

"One implication of our findings is that strain is an important parameter to characterize experimentally," Parker and Soejima said. "The experimentalists making and measuring twisted bilayer graphene do an incredible job juggling and controlling many sources of errors. Eliminating such a small amount of strain is probably terribly tricky, but we suspect someone will work out a way to do it sooner or later."

Overall, the findings suggest that strain is an important 'turning knob' in MATBG as it can elicit phase transitions, thus it should be measured and characterized whenever possible. This observation could have important implications for future research in materials science, as it could help to improve the performance of twisted bilayer graphene.

"Our next goal is to understand the origin of superconductivity in magic-angle graphene," Parker and Soejima said. "One intriguing proposal is that it may be mediated by quasiparticles called Skyrmions instead of the standard phonons. If this is indeed the case, we hope to confirm it by extending the tools used in this work."

© 2021 Science X Network