Study proposes mathematical tool to help understand fractal structure of quark-gluon plasma
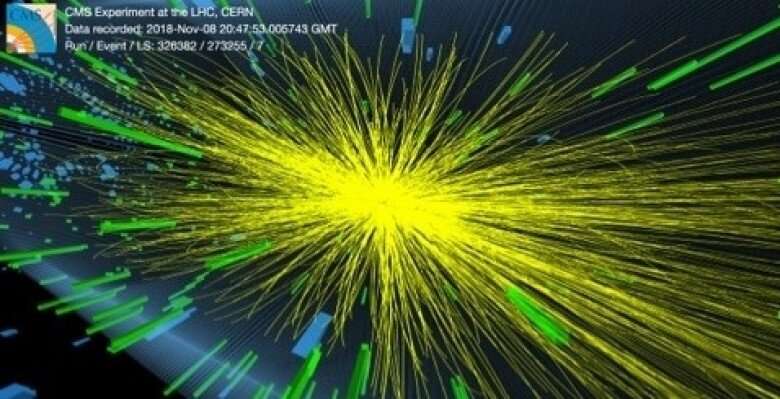
Quark-gluon plasma (QGP) is a state of matter existing at extremely temperatures and densities, such as those that occur in collisions of hadrons (protons, neutrons and mesons). Under so-called "normal" conditions, quarks and gluons are always confined in the structures that constitute hadrons, but when hadrons are accelerated to relativistic velocities and made to collide with each other, as they are in the experiments performed at the Large Hadron Collider (LHC) operated by the European Organization for Nuclear Research (CERN), the confinement is interrupted and the quarks and gluons scatter, forming a plasma. The phenomenon lasts only a tiny fraction of a second, but observation of it has produced important discoveries about the nature of material reality.
One of the discoveries, evidence for which is steadily accumulating, is that quark-gluon plasma has a fractal structure. When it disintegrates into a stream of particles propagating in various directions, the behavior of the particles in the jets is similar to that of the quarks and gluons in the plasma. Moreover, it decays in a cascade of reactions with a pattern of self-similarity over many scales that is typical of fractals.
A new study, published in The European Physical Journal Plus, describes a mathematical tool with which to understand more about the phenomenon. The authors focus on a technical aspect of the solution to the Klein-Gordon equation for the dynamics of bosons, relativistic particles with zero spin that share the same quantum states and are therefore indistinguishable. In a Bose-Einstein condensate (BEC); moreover, particles that behave collectively as if they were a single particle. BEC research has yielded new atomic and optical physics. Potential applications include more accurate atomic clocks and enhanced techniques to make integrated circuits.
"Fractal theory explains BEC formation," said Airton Deppman, a professor at the University of São Paulo's Institute of Physics (IF-USP) in Brazil, and principal investigator for the study.
"The study was part of a broader research program that had already resulted in 2020 in the article 'Fractals, nonextensive statistics, and QCD' published in Physical Review D, demonstrating that Yang-Mills fields have fractal structures and explaining some phenomena seen in high-energy collisions where quark-gluon plasma is formed," Deppman added.
Formulated in the 1950s by Chinese physicist Chen-Ning Yang (joint winner of the 1957 Nobel Prize in Physics) and United States physicist Robert Mills, Yang-Mills theory is highly important to the standard model of particle physics because it describes three of the four fundamental forces in the universe: the electromagnetic, weak and strong forces (the fourth is gravitational interaction).
"In high-energy collisions, the main outcome is particle momentum distributions, which follow Tsallis statistics instead of traditional Boltzmann statistics. We show that the fractal structure is responsible for this. It leads to Tsallis rather than Boltzmann statistics," Deppman continued. Constantino Tsallis was born in Greece in 1943 and became a naturalized Brazilian in 1984. He is a theoretical physicist primarily interested in statistical mechanics. Ludwig Boltzmann (1844-1906) was an Austrian physicist and mathematician who made important advances in statistical mechanics, electromagnetism and thermodynamics.
"With this fractal approach, we were able to determine the Tsallis entropy index q, which is calculated using a simple formula relating it to the key parameters of Yang-Mills," Deppman said. "In the case of quantum chromodynamics [QCD, the theory of the strong interaction between quarks mediated by gluons], these parameters are the number of particle colors and flavors. With these parameters, we found q = 8/7, compatible with experimental results where q = 1.14," he said.
Colors in QCD refer not to the usual concept but to color charges, relating to strong interactions between quarks. There are three possibilities, symbolized by red, green and blue. Quarks also have electric charges, which relate to electromagnetic interactions, but color charges are a different phenomenon. Flavors describe the six types of quark: up, down, charm, strange, top, and bottom. This picturesque nomenclature reflects the sense of humor of Murray Gell-Mann (1929-2019), an American physicist who won the 1969 Nobel Prize in Physics for his work on the theory of elementary particles, and later scientists who also contributed to QCD.
"An interesting aspect of the evolution of our knowledge is that before high-energy collisions were experimentally performed in large particle colliders, and even before the existence of quarks was proposed, Rolf Hagedorn, a German physicist who worked at CERN, set out to predict the production of particles in these collisions," Deppman said. "Solely on the basis of research into cosmic rays, he formulated the concept of fireballs to explain the cascade of particles created in high-energy collisions. With this hypothesis, he predicted the threshold temperature corresponding to the phase transition between confined and deconfined regimes. The key element in his theory is the self-similarity of fireballs. Hagedorn didn't use the term 'fractal' because the concept didn't exist yet, but after the term was coined by Mandelbrot, we saw that fireballs were fractals." Benoît Mandelbrot (1924-2010) was a Polish-born French-American mathematician.
According to Deppman, Hagedorn's theory can be generalized by including Tsallis statistics. Indeed, Deppman did so in an article published in Physica A in 2012.
"With this generalization, we obtain a self-consistent thermodynamic theory that predicts the critical temperature for the transition to quark-gluon plasma, and also supplies a formula for the hadron mass spectrum, from lightest to heaviest," he said. "Strong evidence exists for a conceptual continuity in the description of hadronic systems from quark-gluon plasma to hadrons, and for the validity of the fractal structure of QCD in both regimes."
Deppman questions whether fractal structures could also be present in electromagnetism. This would explain why so many natural phenomena, from lightning to snowflakes, have fractal structures, as they are all governed by electromagnetic forces. It might also explain why Tsallis statistics are present in so many phenomena. "Tsallis statistics have been used to describe scale transformation invariance, a key ingredient of fractals," he said.
Might fractal theory be extended to gravitational phenomena? "Gravitation lies outside the scope of our approach, since it doesn't come into Yang-Mills theory, but there's nothing to stop us speculating whether fractals express an underlying pattern in all material reality," he said.
More information: E. Megías et al, Nonlinear Klein–Gordon equation and the Bose–Einstein condensation, The European Physical Journal Plus (2022). DOI: 10.1140/epjp/s13360-022-02511-2
Journal information: Physical Review D
Provided by FAPESP