Shaking bosons into fermions
Particles can be classified as bosons or fermions. A defining characteristic of a boson is its ability to pile into a single quantum state with other bosons. Fermions are not allowed to do this. One broad impact of fermionic anti-social behavior is that it allows for carbon-based life forms, like us, to exist. If the universe were solely made from bosons, life would certainly not look like it does. Recently, JQI theorists have proposed an elegant method for achieving transmutation—that is, making bosons act like fermions. This work was published in the journal Physical Review Letters.
This transmutation is an example of emergent behavior, specifically what's known as quasiparticle excitations—one of the concepts that make condensed matter systems so interesting. Particles by themselves have mostly well-defined characteristics, but en masse, can work together such that completely distinctive, even exotic phenomena appear. Typically collective behaviors are difficult to study because the large numbers of real particles and all of their interactions are computationally challenging and in many cases prohibitive. JQI Fellow Victor Galitski explains, "The whole idea of emergent excitations is that the quasiparticles are fundamentally different from the actual individual particles. But this actually doesn't happen that often." In this case, it turns out that the boson-to-fermion transmutation leads to an interesting phase of matter. Galitski continues, " Here, the bosons don't condense—they instead form a state without long-range order. This is an example of a long sought after state of matter called a Bose liquid."
In this research, the authors propose a method for realizing and observing such unusual excitations—here the fermionic quasiparticles. The experiment harnesses the strengths of atom-optical systems, such as using bosons (which are easier to work with), a relatively simple lattice geometry (made from lasers that are the workhorses of atomic physics), and established measurement techniques. Galitski continues, "In some sense this was motivated by an experiment where researchers shook a one-dimensional lattice, and it appears that the experiment we propose here is not beyond the capabilities of current work."
Here, the central technique also involves taking an optical lattice made from laser light and shaking it back-and-forth. An atom-optical lattice system, analogous to a crystal, has a periodic structure. Laser beams criss-cross to form standing waves of light that resemble an egg carton. Atoms interact with the light such that they are drawn to the valleys of the egg carton. Like a true solid, this system has an accompanying band-structure, which describes the allowed energies that atoms within the lattice can take on. Without the lattice present, trapped ultracold bosons form a state of matter called a Bose-Einstein condensate. Not much changes when a typical optical lattice is turned on—the bosons will still collect into the lowest energy state and still be in this condensate form. For the simplest lattice configurations, this state corresponds to a single point on a nearly-flattened parabola in the band structure. This configuration is actually the starting point of many atomic physics experiments. Physicists are interested in modifying the energy bands to perhaps uncover more complex phases of matter. To do this, lattice properties must be altered.
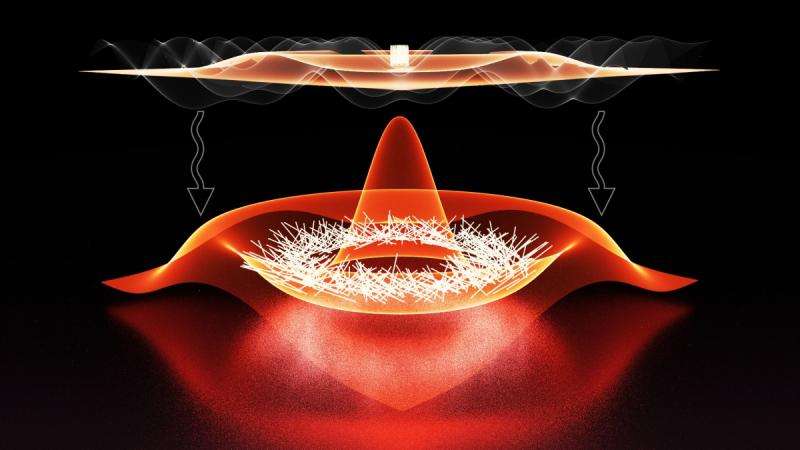
In this work, the authors seek to achieve transmutation, and are among those that have previously shown that one way to accomplish this is to construct a lattice whose band structure looks like a moat. (The word moat here means what it did in medieval times—a trench around a structure.)
Lead author and postdoctoral researcher Tigran Sedrakyan explains the significance of the moat, "The moat is instrumental in achieving this statistical transmutation because it appears that the fermions in a moat-band may actually have lower energy than condensed bosons have, enforcing the constituent bosons to transmute."
It turns out that getting the requisite moat to appear has not been so easy. Surprisingly, in this new work, the team found that if, instead of modifying the lattice geometry itself, they take a simple two-dimensional lattice and shake it back and forth, then a moat appears in what was otherwise an unremarkable, almost flat band structure. The rate of shaking is specially chosen such that the bands undergo this transformation.
The particles themselves do not actually change from bosons to fermions. What's happening is that the environment of the lattice is modifying the bosonic behavior. When the lattice is quivering periodically at a specially determined frequency, the bosons act as if they are governed by fermionic statistics. In the new band structure, the bosons do not form a condensate.
More information: Tigran A. Sedrakyan et al. Statistical Transmutation in Floquet Driven Optical Lattices, Physical Review Letters (2015). DOI: 10.1103/PhysRevLett.115.195301
Journal information: Physical Review Letters
Provided by Joint Quantum Institute