This article has been reviewed according to Science X's editorial process and policies. Editors have highlighted the following attributes while ensuring the content's credibility:
fact-checked
peer-reviewed publication
trusted source
proofread
'Butterfly chaos effect' discovered in swarms and herds of animals
Researchers at the Universidad Carlos III de Madrid (UC3M) and the Universidad Complutense de Madrid (UCM) have discovered a phase shift between chaotic states that can appear in herds of animals and, in particular, in swarms of insects. This advance may help to better understand their behavior or be applied to the study of the movement of cells or tumors.
A phase shift occurs when the conditions of a system change drastically, for example, when water changes from a liquid to a solid state when it freezes. In this research, recently published in the journal Physical Review E, this group of mathematicians has found such a phenomenon in swarms. Related research is also available on the arXiv preprint server.
"The insects in the swarm stay in a limited volume, even if they're in a park or an open space. To explain this, we assume that there is a harmonic potential, a kind of recuperative force that confines them (like that of a spring that tries to return to its resting position when we stretch or contract it)," explains one of the study's authors, Luis L. Bonilla, director of UC3M's Gregorio Millán Barbany Institute.
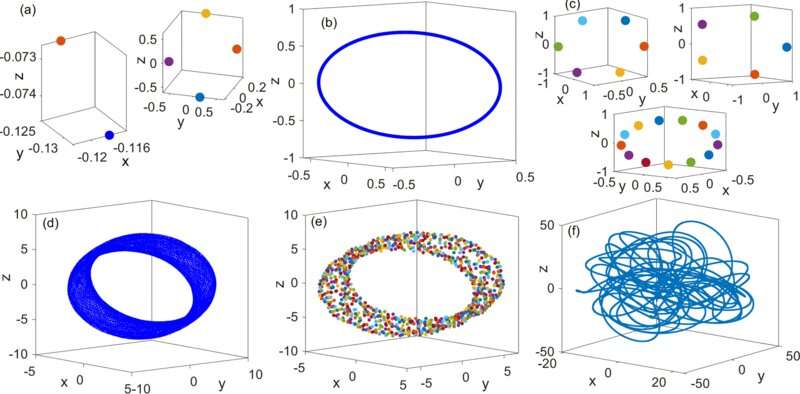
This confinement of the insects responds to a constant of proportionality between force and displacement. Researchers have found that for low confinement values, the movement of the insects in the swarm is chaotic (their movements change a lot if the initial conditions are changed). In this context, the phase shift occurs when the swarm splits into several swarms that are, however, closely related to each other, because there are insects moving from one to another.
At the critical line between phases of this shift, the distance between two insects in the swarm that are influenced by each other is proportional to the size of the swarm, even if the number of insects in the swarm grows indefinitely. This is called "scale-free chaos" and hasn't been discovered until now, according to the researchers.
"As the number of insects increases, the critical line moves towards zero confinement. What happens is that the maximum distance between two insects that still feel each other's influence is proportional to the size of the swarm. It doesn't matter how many insects we put in it. And that represents an absolute novelty that we have discovered," explains Bonilla.
Specifically, what these mathematicians predict through numerical simulations is that certain swarms of insects (specifically a class of small flies) have scale-free chaotic behavior, which translates into certain power laws with exponents similar to those measured in nature. They have also found a simplified mean-field theory that corroborates the scale-free chaos phase shift. "It would be good to look for and find the phase shift between chaotic phases that we predict, either in observations in nature or in controlled laboratory studies," says another of the authors of the research, UCM mathematician Rafael González Albaladejo, who is also linked to UC3M's Gregorio Millán Barbany Institute.
The formation of herds is one of the manifestations of so-called "active matter," made up of something like self-propelled individuals that form a whole, the researchers explain. It can be a swarm of insects, a flock of sheep, a flock of birds, a school of fish, but also bacteria in motion, melanocytes (the cells that distribute pigments in the skin) or artificial systems such as periodically shaken irregular grains or seeds. "Herd formation mechanisms play a role in some of these systems, so the results we have obtained can be linked to biology, to the study of cells, and beyond that, to the study of tumors and other diseases," adds Albaladejo.
How do so many animals move in unison? These researchers explain that each individual only senses its neighbors and moves accordingly, even though it has no perspective on the movement of the whole herd. And depending on whether they use sight, hearing or the vibrations of the fluid in which they are immersed, the concept of neighbor can change quite a bit.
Sheep moving together see and sense those around them, while birds in a flock see their nearest neighbors, even if they are quite far apart. "Moving accordingly may mean that they move in the same direction as their neighbors (the norm) or they may adopt different strategies depending on the situation. For example, if a crowd is trying to get out of a crowded pen with more than one gate, there are times when not following neighbors is advantageous," they explain.
It has taken the mathematicians about two years to carry out this research work. Initially, they set out to explain experiments by studying the conventional phase shift between a crowd of insects that fill a space with constant density and become ordered when passing a critical value of the control parameter (e.g., by decreasing the noise). But then they decided to add a harmonic potential to confine the swarm and explore what happens when the attractive force between individuals decreases.
"We discovered many periodic, quasi-periodic and finally chaotic states for a fixed number of insects that we increased. The surprising thing is the transition between chaotic states that we didn't know or assume existed, and we were able to find the correct arguments and tests to support their existence," says another of the study's authors, Ana Carpio, from UCM's Department of Mathematical Analysis and Applied Mathematics, who points out that there is still a lot to be done based on this work.
"From experimentally seeking confirmation of our predictions and better adapting the model to experimental observations, to carrying out theoretical and mathematical research that goes beyond our numerical simulations," she concludes.
More information: R. González-Albaladejo et al, Scale-free chaos in the confined Vicsek flocking model, Physical Review E (2023). DOI: 10.1103/PhysRevE.107.014209
R. González-Albaladejo, L. L. Bonilla, Mean field theory of chaotic insect swarms, arXiv (2023). arxiv.org/abs/2305.14085
Journal information: Physical Review E , arXiv
Provided by Carlos III University of Madrid