Realization of photonic p-orbital higher-order topological insulators

In condensed matter systems, an essential characteristic of electrons besides charge and spin is the orbital degree of freedom (ODoF). It plays a crucial role in understanding unconventional properties in solid-state materials and in "orbital physics" toward unveiling the science and technology of correlated electrons. However, due to the complexity and various degrees of freedom simultaneously involved in real materials, it has always been a challenge to fully unravel the physics of strongly correlated electronic matter mediated by the ODoF via controlled experiments.

The interest in synthetic orbital systems, including trapped atoms in optical lattices, surged rapidly over the past decade, leading to "orbital-only" quantum emulators for fermions and bosons. Orbital lattices have been employed to demonstrate complex Bose-Einstein condensates, orbital superfluidity, and exotic topological semimetal phases. The capability of purposely preparing atoms to higher orbital bands in optical lattices has opened the door for understanding orbital physics in condensed matter systems, with the ultimate goal of exploring new quantum states of matter not found in natural solids.

In a new paper published in eLight, a team of scientists led by Professor Zhigang Chen from Nankai University and Professor Hrvoje Buljan from the University of Zagreb have found a new pathway to explore novel orbital phenomena mediated by higher band topology.

Other synthetic platforms have emerged and caught increasing attention. They include polaritonic semiconductor lattices, laser-written photonic lattices, nanomechanical resonant structures, and atom-to-atom engineered electronic lattices. In particular, by employing the ?-orbital bands of polariton micropillars arranged in a honeycomb lattice, both orbital edge states and exotic Dirac dispersions were observed. These artificial lattices can flexibly manipulate orbital Dirac matter with transport properties and topological features difficult to achieve in conventional solid-state systems.

Symmetry-protected topological (SPT) phases have been extensively explored due to their peculiar characteristics and unique application potential associated with robust boundary states, especially in photonics. Notable recent endeavors have focused on realizing higher-order topological insulators (HOTIs). HOTIs are a new topological material class that does not obey the conventional bulk-edge correspondence principle. Their underlying physics is still an ongoing subject of investigation.

Higher-order topological corner states have been extensively tested for various applications, including topological nanocavities and lasers. However, to date, all experimental studies in HOTI systems relied on the ?- orbital band, leaving higher-orbital HOTIs unexplored except for a recent theoretical proposal.

As an exciting and intriguing model of HOTIs, the breathing Kagome lattices (BKLs) exhibiting ?3 rotational symmetry have been widely explored to demonstrate ?-band HOTIs in many different systems. There is a continuing debate concerning the HOTI classification for the BKLs. Experiments using metamaterials have unambiguously identified the higher-order topology for the ?-orbital BKL-type topological crystalline insulators.

Furthermore, their robustness to symmetry-breaking perturbations has also been validated. It is thus natural to ask: can we realize a HOTI manifesting higher orbitals using a synthetic BKL platform, and what are the distinctive topological features and new perspectives associated with the orbital HOTIs?

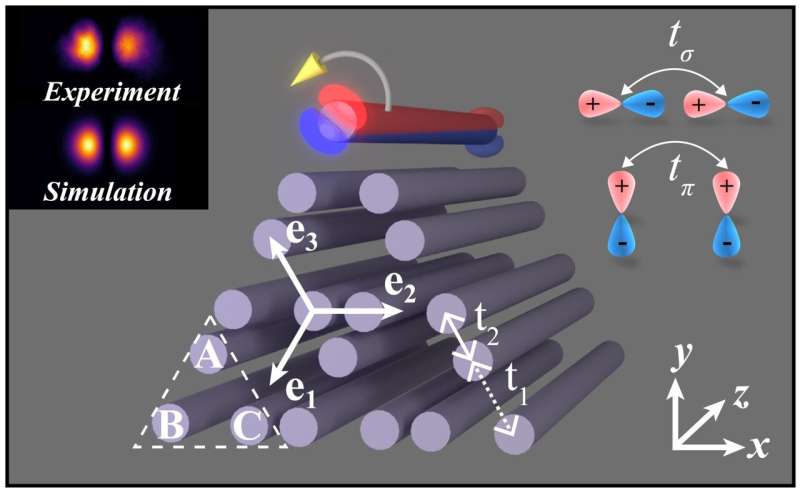
The researchers have experimentally demonstrated ?-orbital HOTIs using photonic BKLs established by a continuous-wave (CW) laser-writing technique. In a triangle-shaped nontrivial BKL, both ??- and ??-type orbital corner states are observed. They displayed characteristic intensity and phase structures manifesting the zero-dimensional "zero-energy" modes. Corner excitation leads to the dynamical rotation of a dipole beam due to the nonlinearity-induced lifting of ?? and ?? orbital mode degeneracy.

The researchers calculated the band structures and topological invariants of the orbital HOTIs and confirmed their nontrivial topology from the winding number. However, the topology is "hidden" in the conventional bulk polarization due to orbital coupling-induced band crossing. The SPT phase of orbital HOTIs is found to be inherited from the lower ?- band Hamiltonian, being protected by the ?3 rotational symmetry, the generalized chiral symmetry (GCS), as well as by a previously unknown orbital-hopping symmetry not applicable to the ?-band HOTIs.

The ODoF requires each waveguide in the BKL to support at least the higher orbital ? -mode. The fs-laser-writing BKL waveguide, used for the ?—orbital HOTI, supports only a fundamental ?-mode. Different from the ?- orbital, the coupling strength of the ?-orbital is orientation-dependent, so one needs two types of coupling parameters ?_? and ?_?, to describe the orbital coupling dynamics.

For the ? -orbital HOTI, the conventional bulk polarization can be used to characterize the topological properties of the corner states. The researchers have shown that the nontrivial topology of the ? -orbital HOTI is "hidden" if the bulk polarization is used due to hybridization effects. However, they are well manifested by the generalized winding number. The researchers have found that the topological protection of ? -orbital corner states demands an orbital-hopping symmetry, which has no counterpart in the ?-orbital HOTI.