December 18, 2022 feature
Study uncovers existing limitations in the detection of entanglement
Quantum entanglement is a process through which two particles become entangled and remain connected over time, even when separated by large distances. Detecting this phenomenon is of crucial importance for both the development of quantum technology and the study of quantum many-body physics.
Researchers at Tsinghua have recently carried out a study exploring the possible reasons why the reliable and efficient detection of entanglement in complex and "noisy" systems has often proved to be very challenging. Their findings, published in Physical Review Letters, hint at the existence of a trade-off between the effectiveness and efficiency of entanglement detection methods.
"Over 20 years ago, researchers discovered that most quantum states are entangled," Xiongfeng Ma, one of the researchers who carried out the study, told Phys.org.
"This means that, for example, if we managed to construct a 100-qubit system, say, a superconducting or ion-trap quantum computing system, this system will evolve for a while, during which the qubits extensively interact with each other. Of course, there will be errors, so to maintain a good coherent control, we reasonably isolate the system from the environment. As long as the purity (quantifying the effectiveness of our isolation effort) is not exponentially small with the number of qubits, the system is highly likely to be entangled."
While entanglement might theoretically seem fairly simple to realize, achieving it in experimental settings is in fact very difficult. Studies showed that it is particularly difficult in large quantum systems, such as systems comprised of 18-qubits. The key objective of the recent work by Ma and his colleagues was to gain a better understanding of the challenges associated with the detection of entanglement in large systems.
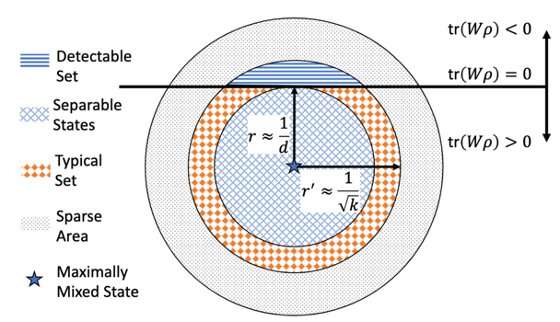
"Researchers gradually realized that while the preparation of entangled state for a large system might be easy, the entanglement detection could be very challenging in practice," Ma explained. "In our work, we establish a mathematical formulation to quantify the effectiveness of an entanglement detection method. We employ a proper quantum state distribution, use the ratio of detectable entangled state to quantify its effectiveness, and also quantify the efficiency of an entanglement detection method by the number of observables needed for this method."
Ma and his colleagues first examined what is arguably the most straightforward entanglement detection protocol available today, known as entanglement witnesses. They showed that this protocol's ability to detect entanglement decreases by a double exponential value as the system gets larger.
The researchers later found that this reduction in effectiveness linked to a system's size also affected other entanglement detection protocols. After a series of theoretical considerations, they were able to extend their observations of the entanglement witnesses method's performance to arbitrary entanglement protocols that rely on single-copy quantum state measurements.
"For a random state coupled with the environment, any entanglement detection protocol with single-copy realization is either inefficient or ineffective," Ma said. "Inefficient means the protocol relies on measuring an exponential number of observables and ineffective means the success rate of entanglement is double exponentially low."
Essentially, Ma and his colleagues showed that to observe entanglement on a large-scale, researchers must be able to control all interactions in a system with high precision and know almost all information about them. When there is a lot of uncertainty about the system, therefore, the probability of detecting its entanglement is very small, even if one is almost certain of its occurrence.
"We proved that no entanglement detection protocols are both efficient and effective," Ma explained. "This may help the design of entanglement detection protocols in the future. Meanwhile, detecting large-scale entanglement could be a good indicator for comparing different quantum computing systems. For example, when a lab team claim they build a hundreds-of-qubit system, they should detect entanglement. Otherwise, they have not controlled the system well enough."
Overall, the findings gathered by this team of researchers highlight the existence of a trade-off in the efficiency and effectiveness of existing entanglement detection methods. In addition, they offer valuable insight about the reasons why detecting entanglement in large-scale and noisy quantum systems is so difficult.
"Our result does not prevent us from designing a protocol that is both efficient and effective when the system is well-controlled (i.e., the coupled environment is relatively small)," Ma added. "Currently, we only have entanglement detection protocols that work well for pure states, such as entanglement witnesses, and protocols that work for large environments at the expense of exponential cost. We noticed that an entanglement detection protocol that works for moderate environment size with relatively low cost is still missing, and we would now like to try to develop one."
More information: Pengyu Liu et al, Fundamental Limitation on the Detectability of Entanglement, Physical Review Letters (2022). DOI: 10.1103/PhysRevLett.129.230503
Karol Życzkowski et al, Volume of the set of separable states, Physical Review A (2002). DOI: 10.1103/PhysRevA.58.883
Leonid Gurvits et al, Largest separable balls around the maximally mixed bipartite quantum state, Physical Review A (2002). DOI: 10.1103/PhysRevA.66.062311
Stanislaw J. Szarek, Volume of separable states is super-doubly-exponentially small in the number of qubits, Physical Review A (2005). DOI: 10.1103/PhysRevA.72.032304
Xi-Lin Wang et al, 18-Qubit Entanglement with Six Photons' Three Degrees of Freedom, Physical Review Letters (2018). DOI: 10.1103/PhysRevLett.120.260502
Journal information: Physical Review Letters , Physical Review A
© 2022 Science X Network