Topological superconducting phase protected by 1-D local magnetic symmetries

Topological superconductors (TSCs) are new kind of topological quantum states with fully superconducting gapped band structure in the bulk, but they support gapless excitations called Majorana zero modes (MZMs) at the boundaries. Because of their nonlocal correlation and non-Abelian statistic nature, MZMs are proposed as the qubits of topological quantum computation. Hence, searching and operating the MZMs in TSC materials is now an important topic in condensed matter physics.
To identify a TSC, one should first ascertain its topological classification. The topological classification highly depends on the symmetries including time-reversal symmetry, particle-hole symmetry, and especially the crystalline symmetries. Without the consideration of crystalline symmetries, the Bogoliubov-deGennes (BdG) Hamiltonians of the 1-D superconductors only have the Z2 classification. The mirror reflection symmetry and rotational symmetries can enhance the classification to Z class. Nevertheless, the topological classification of superconductors with general magnetic symmetries is still an open question.
In a new research article published in the Beijing-based National Science Review, scientists from the Huazhong University of Science and Technology in Wuhan, China, and Princeton University in New Jersey, USA proposed the method to classify the topological superconducting phase by examining the compatibility between different MZMs. Co-authors Jinyu Zou, Qing Xie, Zhida Song and Gang Xu analyzed the topological classification of gapped superconducting wires with local magnetic symmetries (LMSs). They found that an effective BDI class TSC can be realized in MxT or C2zT invariant wire. Remarkably, the new TSC phases characterized by Zh invariant in C4zT case and Zhoplus Zc invariant in C6zT case are discovered.

In the article titled "New types of topological superconductors under local magnetic symmetries". The authors focus on the 1D superconducting wires with LMSs T'= MxT, C2zT, C4zT and C6zT. "The operation of T' does not change the position of electrons. Hence it acts on the BdG Hamiltonian like a time-reversal operator". Combining T' and particle-hole symmetry P leads to a chiral symmetry S = T'P. The BdG Hamiltonian can adopt the diagonalized form according to the chiral symmetry. And the MZMs are the eigenstates of the chiral symmetry S. The authors find "MZMs having chiral eigenvalues s and -s can couple to each other and be eliminated." Following the guideline, they analysis the compatibility of the MZMs at the end of the 1D superconducting wires with LMSs, and summarize their topological classification as listed in Table I.
The MxT and C2zT cases are equivalent to the BDI class with chiral topological invariant Zc. While C4zT case is characterized by helical Zh invariant, which indicate multiple Majorana Kramer pairs at the end of the superconducting wire. In the C6zT case, "the topology of the whole BdG Hamiltonian is classified by Zh oplus Zc,". In such a novel topological phase, "the helical and chiral MZMs can coexist."
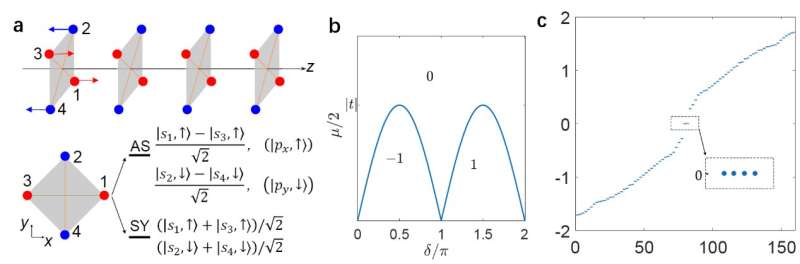
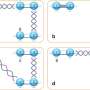
"To illustrate the TSC phase with the LMS C4zT, we construct a 1D anti-ferromagnetic chain along z-direction," the scientists add. They give the topological phase diagram of the model. "In the nontrivial TSC phase, the open quantum wire traps an integer pairs of MZMs at its ends." they also show the MZMs through numerical and analytical calculation.
"These results not only enrich the variety of the 1-D TSC, but also provide luxuriant building blocks for the construction of new type 2-D and 3-D TSCs" they forecast at the end of the article, "For example, one can couple the 1D TSCs in y direction to construct a 2-D TSC. The high symmetry lines ky = 0 and ky =pi in momentum space preserve the 1D LMS. With proper parameters, the ky = 0 and ky =pi lines can belong to distinct topological phase, and result in the gapless propagating Majorana edge states connecting the conducting bands and valence bands."
More information: Jinyu Zou et al, New types of topological superconductors under local magnetic symmetries, National Science Review (2020). DOI: 10.1093/nsr/nwaa169
Provided by Science China Press