Mathematicians unravel a thread of string theory
Simply put, string theory is a proposed method of explaining everything. Actually, there's nothing simple about it. String theory is a theoretical framework from physics that describes one-dimensional, vibrating fibrous objects called "strings," which propagate through space and interact with each other. Piece by piece, energetic minds are discovering and deciphering fundamental strings of the physical universe using mathematical models. Among these intrepid explorers are Utah State University mathematicians Thomas Hill and his faculty mentor, Andreas Malmendier.
With colleague Adrian Clingher of the University of Missouri-St. Louis, the team published findings about two branches of string theory in the paper, "The Duality Between F-theory and the Heterotic String in D=8 with Two Wilson Lines," in the August 7, 2020 online edition of Letters in Mathematical Physics. The USU researchers' work is supported by a grant from the Simons Foundation.
"We studied a special family of K3 surfaces—compact, connected complex surfaces of dimension 2—which are important geometric tools for understanding symmetries of physical theories," says Hill, who graduated from USU's Honors Program with a bachelor's degree in mathematics in 2018 and completed a master's degree in mathematics this past spring. "In this case, we were examining a string duality between F-theory and heterotic string theory in eight dimensions."
Hill says the team proved the K3 surfaces they investigated admit four unique ways to slice the surfaces as Jacobian elliptic fibrations, formations of torus-shaped fibers. The researchers constructed explicit equations for each of these fibrations.
"An important part of this research involves identifying certain geometric building blocks, called 'divisors," within each K3 surface," he says. "Using these divisors, crucial geometric information is then encoded in an abstract graph."
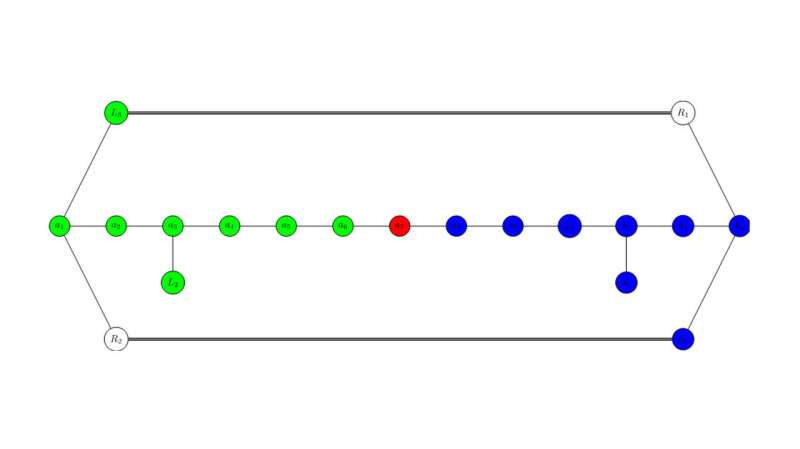
This process, Hill says, enables researchers to investigate symmetries of underlying physical theories demonstrated by the graph.
"You can think of this family of surfaces as a loaf of bread and each fibration as a 'slice' of that loaf," says Malmendier, associate professor in USU's Department of Mathematics and Statistics. "By examining the sequence of slices, we can visualize, and better understand, the entire loaf."
The undertaking described in the paper, he says, represents hours of painstaking "paper and pencil" work to prove theorems of each of the four fibrations, followed by pushing each theorem through difficult algebraic formulas.
"For the latter part of this process, we used Maple Software and the specialized Differential Geometry Package developed at USU, which streamlined our computational efforts," Malmendier says.
More information: Adrian Clingher et al. The duality between F-theory and the heterotic string in D=8 with two Wilson lines, Letters in Mathematical Physics (2020). DOI: 10.1007/s11005-020-01323-8
Provided by Utah State University