Mathematician proposes a new criterion for solving the Boussinesq equations
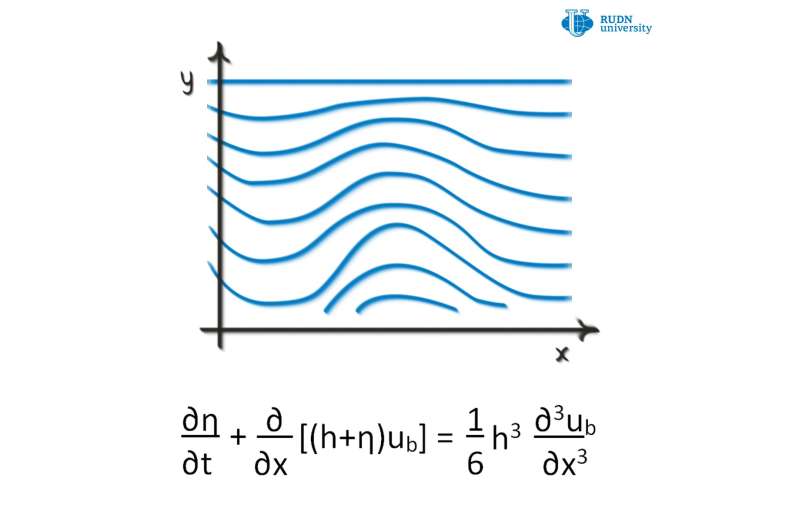
A RUDN University mathematician has proposed a new criterion for solving the Boussinesq equations. These equations describe the nonlinear propagation of waves in certain media, e.g. plasma, a surface of liquid of shallow depth, and so on. They examined the Boussinesq equation in three-dimensional space and derived a criterion for uniqueness and the existence of important solutions of a special type to the Boussinesq partial differential equation. The proposed criterion has applications in mechanics of continuous media, which studies the motion of liquids and gases. The article was published in Bulletin of the Brazilian Mathematical Society, New Series.
Both the Boussinesq equations and the Navier-Stokes equations are systems of partial differential equations (differentiation is carried out with respect to all independent variables). Partial differential equations play a significant role in mathematical physics and mechanics. Solving equations of this type is often fraught with great difficulties. The problem of existence and uniqueness of a solution to the Boussinesq equations under given initial conditions (the so-called Cauchy problem) had previously been investigated by many scientists, including the authors of the article. With certain values of the parameters, the Boussinesq equations turn into Navier-Stokes equations. The existence and continuous differentiability, or, as mathematicians say, smoothness, of solutions to the Navier-Stokes equations is one of the seven Millennium Prize Problems, posed in 2000 by the Clay Mathematics Institute.
For some functional spaces (namely, for homogeneous Besov spaces, pf which the famous Sobolev spaces are a special case), the problem has been successfully solved by mathematicians Don and Zhang. RUDN University mathematician Maria Alessandra Ragusa and her colleague went further, proving a similar criterion for the Boussinesq equations in homogeneous Besov spaces. The authors examined the Boussinesq equations in three-dimensional space, which makes it possible to more fully apply the results in the natural sciences.
Having introduced a number of necessary definitions and proved auxiliary lemmas, the author from RUDN University successfully proved the main theorem and showed that the solution to the Cauchy problem not only exists, is unique, and does not have singular points, but also smoothly extends to a larger interval of an independent variable. The article uses the apparatus of functional analysis, a mathematical discipline with a high level of abstraction. Nevertheless, such results can find wide and fruitful application in mechanics and physics.
More information:
A Regularity Criterion of Weak Solutions to the 3-D Boussinesq Equations.
Bulletin of the Brazilian Mathematical Society, New Series, 31 July 2019, link.springer.com/article/10.1007%2Fs00574-019-00162-z
Provided by RUDN University