May 17, 2016 report
Deep space experiment could measure the gravitational constant with nearly 1,000 times improvement in accuracy (Update)
(Phys.org)—Scientists have proposed an experiment that could measure the value of Newton's gravitational constant, G, from deep space instead of an Earth-based laboratory. The researchers predict that the deep space experiment could estimate G with an improvement in precision of nearly three orders of magnitude, since it would avoid the influence of Earth's gravity.
The researchers, Michael Feldman et al., have published a paper on the proposed experiment in a recent issue of Classical and Quantum Gravity.
Uncertainty with Big G
Newton's gravitational constant, G, determines the strength of the gravitational force between any two objects anywhere in the universe. Over the past century, a dozen or so Earth-based experiments have used torsion balances, atom interferometers, and other tools to measure the value of G to be approximately 6.67408 x 10-11, with an uncertainty of 4.7 × 10−5.
Although this may sound precise, it is not very precise at all compared to many other physical constants, which have uncertainties that are many orders of magnitude smaller than this. In recent years, the large variations in the measured values of G have caused scientists to question if G is truly constant at all. (Currently, the overwhelming consensus is that G is constant, and that the variations are due to large systematic measurement errors.)
"G is currently the least well known of all the fundamental physical constants, which is embarrassing," Feldman told Phys.org. "A more precise number, and the possibility that G could vary with time, location, or the type of matter involved, could link to improvements in Einstein's general relativity, including quantum gravity."
One of the main reasons that G is so difficult to measure accurately is that experiments must account for the influence of Earth's gravity, g (sometimes called "little g" in contrast to "big G"). Little g is the acceleration due to gravity specifically on Earth, where it has a constant value of approximately 9.8 m/s2. Elsewhere in the universe, this value changes, since it depends on the Earth's mass and the distance between the Earth and another object. However, the value of big G does not depend on these factors, and so it remains the same everywhere in the universe.
Deep space lab
In the new paper, the researchers suggest that the best way to avoid the effects of Earth's gravity on measurements of G is to perform the experiment in deep space, which refers to space outside our solar system.
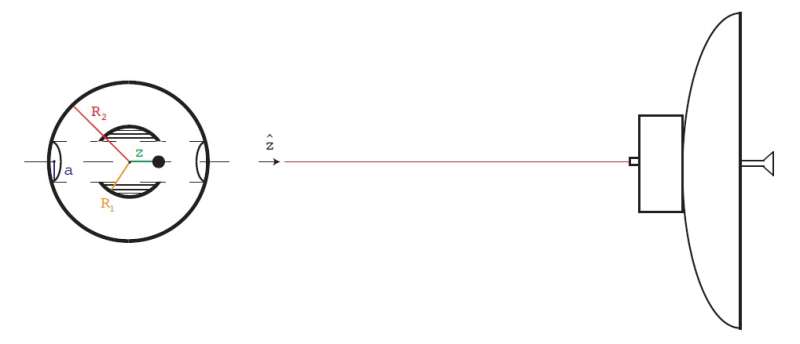
The scientists propose to launch their apparatus into deep space, likely by "piggybacking" on a major mission. Out there, where the gravity of planets and stars would be negligible, the host spacecraft would release a spherical object that has a 1-cm-wide tunnel through its center. Then (this would likely be the most difficult part), the host spacecraft—which is constantly spinning the whole time—would eject a much smaller oscillating object into the tunnel in the sphere at just the right angle and speed so that the object would move back and forth through the tunnel, without bouncing off the walls.
The host apparatus would continually shine femtosecond laser pulses on the object as it oscillates in the tunnel, and the object (a retroreflector) would reflect these pulses back to the host spacecraft. These pulses would provide data on the period of the object's harmonic motion, which is directly dependent on the value of G. The data would then be sent back to Earth via radio communication for interpretation.
If everything goes as expected, the researchers' simulations showed that this experiment could measure G with an uncertainty of 6.3 x 10-8, which is nearly three orders of magnitude more precise than the current best measurement.
Even though the deep-space experiment wouldn't have to deal with the Earth's gravity, it would still have to contend with other, smaller non-gravitational accelerations that would also affect the retroreflector's motion. These influences include solar radiation pressure, solar tidal effects, cosmic rays, and the momentum from the laser pulses. Some of these effects could be dealt with through careful design—for example, the sphere could be shielded from solar radiation pressure by positioning it in the shadow of the host spacecraft. But the researchers explain that any acceleration greater than 10-17 m/s2 must be modeled and accounted for when interpreting the data.
Why measure G?
The National Science Foundation in the US recently issued a solicitation for new approaches for measuring G (Ideas Lab: Measuring "Big G" Challenge). The NSF webpage says that measuring a more precise value of G will benefit many fields of physics and metrology, such as understanding the Casimir effect, improving the spring constants that are used to calibrate atomic force microscopy cantilevers, and understanding intermolecular forces in DNA. A precise value of G might also be used to test proposed theories that unify gravity with quantum electrodynamics.
More information:
Michael R. Feldman et al. "Deep space experiment to measure G." Classical and Quantum Gravity. DOI: 10.1088/0264-9381/33/12/125013
Also at arXiv:1605.02126 [gr-qc]
© 2016 Phys.org