Scientists develop a semi-device independent, randomness-free test for quantum correlation

In a new Physical Review Letters study, scientists have successfully presented a proof of concept to demonstrate a randomness-free test for quantum correlations and non-projective measurements, offering a groundbreaking alternative to traditional quantum tests that rely on random inputs.

"Quantum correlation" is a fundamental phenomenon in quantum mechanics and one that is central to quantum applications like communication, cryptography, computing, and information processing.

Bell's inequality, or Bell's theory, named after physicist John Stewart Bell, is the standard test used to determine the nature of correlation. However, one of the challenges with using Bell's theorem is the requirement of seed randomness for selecting measurement settings.

In other words, the inputs for the experiment need to be truly random, which is the challenge. Additionally, seed randomness can be expensive and vulnerable to loopholes.

The new study, led by Dr. Jacquiline Romero from the University of Queensland and the Australian Research Council Center of Excellence for Engineered Quantum Systems, eliminates the need for this seed randomness by proposing an alternate test.

Dr. Romero explained this to Phys.org, saying, "Our work does away with this stringent requirement for randomness. We demonstrate that the shared (or correlated) randomness acquired from entangled coins cannot be replicated using two two-level classically correlated coins. This discovery empowers us to establish a quantum advantage in the toy game described in our paper."

She also expressed her enthusiasm for this research, saying, "I am always on the lookout for experiments that highlight the difference between classical and quantum information because these experiments spark curiosity."

Bell's inequality and device independence

Real-life implementation of quantum systems and protocols is challenging due to many reasons. One of the main challenges is the need for idealized modeling and a detailed understanding of all its parts. Without such knowledge, these protocols become vulnerable to various threats.

However, in reality, we don't have all the information about the quantum system. Co-author of the study, Dr. Manik Banik from the S.N. Bose National Center for Basic Sciences in India, explained, "In practice, Bell's inequality serves as a crucial tool to certify non-classicality in a 'device-independent' manner, enabling fully device-independent protocols without detailed knowledge of quantum device operations."

"However, practical scenarios often involve partial knowledge about device characteristics, leading to semi-device independence."

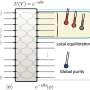
In these situations, we possess some information about the quantum system, such as the dimensions of the subsystems involved, but not a complete understanding of its inner workings. This is precisely what the team did.

"We propose a solution to this non-classicality certification task from output statistics only, but additional information about the internal working of the device is required namely the operational dimension. This additional albeit minimal knowledge about the device deems the technique a semi-device independent status," explained other co-author, Some Sankar Bhattacharya, from the University of Gdansk, Poland.

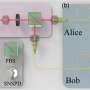
Entangled photons, Alice & Bob

The team's experimental setup hinged on the production of entangled photons using a non-linear crystal through a process known as spontaneous parametric down-conversion (SPDC).

In essence, the SPDC process in a non-linear crystal takes the high-energy pump photons, absorbs them, and spontaneously generates pairs of lower-energy entangled photons.

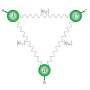
The entangled photons were then randomly sent to the two parties, Alice and Bob, using a beam splitter. Alice and Bob measured the spatial modes of the photons, which is a property describing how the photons are distributed in space.

To make the measurements on the entangled photons, Alice and Bob used qubit trine positive operator-valued measures or POVMs, which are a set of measurement operators representing non-projective measurements.

Non-projective measurements are quantum measurements that go beyond standard projective measurements, allowing for a more comprehensive characterization of quantum systems.

Next, the team recorded outputs every time there was a correlated outcome between Alice and Bob. They then performed calculations to determine joint probability distributions, which allowed them to assess the probability of obtaining specific measurement outcomes that were correlated between Alice and Bob.

For example, if they were playing a game with the entangled photons and measuring whether they both got head (H) or tails (T), a joint probability distribution would tell them the likelihood of both getting H, both getting T, or one getting H and the other getting T.

The setup is semi-device independent because the only known variables were the input (entangled photons) and output (measurements).

Quantum advantage and shared randomness

In the realm of quantum systems, the notion of a quantum advantage challenges classical notions of randomness. In this experiment, it means demonstrating shared randomness.

Classical systems, like coin tossing, assign predetermined probabilities to each possible outcome. For example, a fair coin has an equal 50% chance of landing on either H or T in each toss. However, in a quantum system, we see correlated outcomes that appear entirely random but are fundamentally entangled.

Imagine a scenario where Alice and Bob independently toss their respective coins. Remarkably, the outcomes of their coin tosses are mysteriously intertwined. When Alice gets a H, Bob simultaneously gets a H, and when Alice gets a T, Bob also gets a T.

This shared randomness is established through quantum entanglement, where particles become interconnected, and their properties remain correlated regardless of physical separation.

The team demonstrated quantum advantage through their experiment by showing that the correlated coin obtained from the entangled photons cannot be replicated using two two-level classical correlated coins.

Dr. Romero explained its implications for quantum information processing, "Shared (or correlated) randomness is a useful resource for many tasks."

"Quantum communication protocols, such as certain secret-sharing schemes or quantum computations involving a randomness distribution component (which has been shown to enhance security), stand to benefit from our results."

For future studies, she hopes to explore the possibility of making quantum advantage device-independent and demonstrating it experimentally.

© 2023 Science X Network