Researchers investigate properties of double white dwarfs with new mass transfer stability criterion

White dwarfs comprise a class of faint stars representing the endpoint of the evolution of intermediate- and low-mass stars. The mass of a white dwarf is the order of that of the sun, but a radius comparable to that of the Earth. A pair of white dwarfs in orbit around their common center of gravity is called a double white dwarf (DWD).

Researchers led by Dr. Li Zhenwei from the Yunnan Observatories of the Chinese Academy of Sciences studied the influence of mass transfer stability criterion on the formation and properties of DWD populations in the galaxy.

This work was published in Astronomy & Astrophysics on Jan. 13.

In general, a binary will experience at least one mass transfer phase to produce a DWD. If the mass transfer is stable, the donor star can transfer mass to the companion on a long timescale. On the contrary, the unstable mass transfer proceeds on a dynamical timescale, and the binary would enter into the common envelope phase.

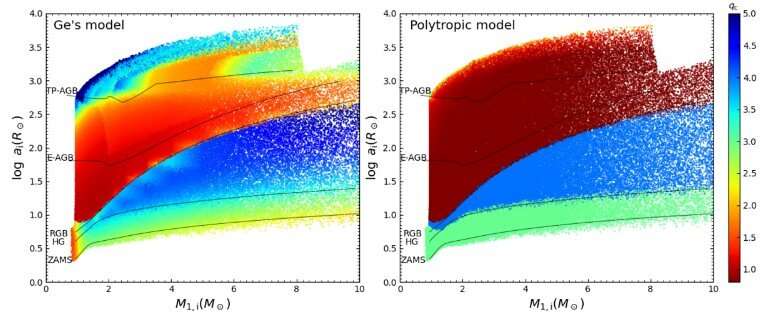
Whether or not the mass transfer is stable is mainly determined by the mass transfer stability criterion. However, previous mass transfer stability criterion based on polytropic models cannot reproduce the orbital parameters of some observed DWDs.

Ge et al. obtained the new mass transfer stability criterion by adopting the adiabatic mass loss model, or Ge's model. In this study, the researchers performed a series of binary population synthesis with Ge's model, and confirmed that mass transfer stability criterion played an important role in the formation and properties of DWD populations.

Comparing findings with the previous results from the polytropic model, they found that the merger rate distribution in the observations could be well reproduced in Ge's model without any tuning of parameters. The results from Ge's model support the observations better than what can be derived from the polytropic model.

In addition, the researchers calculated the number of detectable gravitational wave sources in Ge's model, and the results provide theoretical supports for future space-based gravitational wave detections.