Quantum hall effect 'reincarnated' in 3-D topological materials
U.S. and German physicists have found surprising evidence that one of the most famous phenomena in modern physics—the quantum Hall effect—is "reincarnated" in topological superconductors that could be used to build fault-tolerant quantum computers.
The 1980 discovery of the quantum Hall effect kicked off the study of topological orders, electronic states with "protected" patterns of long-range quantum entanglement that are remarkably robust. The stability of these protected states is extremely attractive for quantum computing, which uses quantum entanglement to store and process information.
In a study published online this month in Physical Review X (PRX), theoretical physicists from Rice University, the University of California, Berkeley (UC Berkeley), and the Karlsruhe Institute of Technology (KIT) in Karlsruhe, Germany, presented strong numerical evidence for a surprising link between 2-D and 3-D phases of topological matter. The quantum Hall effect was discovered in 2-D materials, and laboratories worldwide are in a race to make 3-D topological superconductors for quantum computing.
"In this work we've shown that a particular class of 3-D topological superconductors should exhibit 'energy stacks' of 2-D electronic states at their surfaces," said Rice co-author Matthew Foster, an associate professor of physics and astronomy and member of the Rice Center for Quantum Materials (RCQM). "Each of these stacked states is a robust 'reincarnation' of a single, very special state that occurs in the 2-D quantum Hall effect."
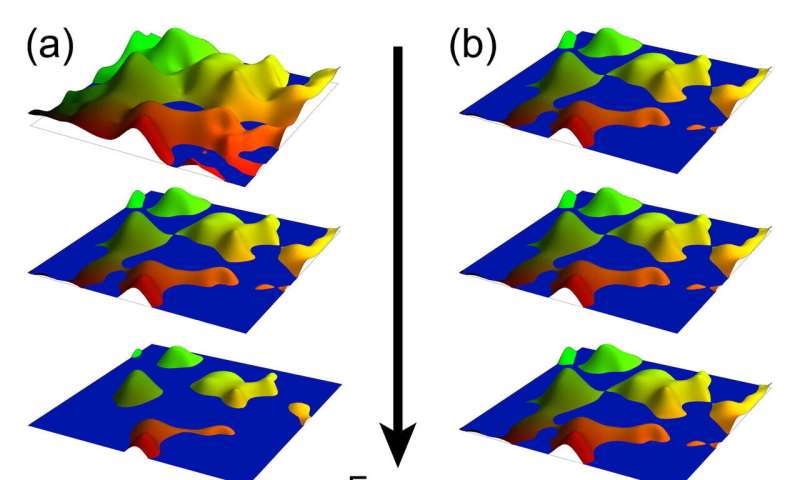
The quantum Hall effect was first measured in two-dimensional materials. Foster uses a "percolation" analogy to help visualize the strange similarities between what occurs in 2-D quantum Hall experiments and the study's 3-D computational models.
"Picture a sheet of paper with a map of rugged peaks and valleys, and then imagine what happens as you fill that landscape with water," he said. "The water is our electrons, and when the level of fluid is low, you just have isolated lakes of electrons. The lakes are disconnected from one another, and the electrons can't conduct across the bulk. If water level is high, you have isolated islands, and in this case the islands are like the electrons, and you also don't get bulk conduction."
In Foster's analogy the rugged landscape is the electric potential of the 2-D material, and the level of ruggedness corresponds to amount of impurities in the system. The water level represents the "Fermi energy," a concept in physics that refers to the filling level of electrons in a system. The edges of the paper map are analogous to the 1D edges that surround the 2-D material.
"If you add water and tune the fluid level precisely to the point where you have little bridges of water connecting the lakes and little bridges of land connecting the islands, then it's as easy to travel by water or land," Foster said. "That is the percolation threshold, which corresponds to the transition between topological states in quantum Hall. This is the special 2-D state in quantum Hall.
"If you increase the fluid level more, now the electrons are trapped in isolated islands, and you'd think, 'Well, I have the same situation I had before, with no conduction.' But, at the special transition, one of the electronic states has peeled away to the edge. Adding more fluid doesn't remove the edge state, which can go around the whole sample, and nothing can stop it."
The analogy describes the relationship between robust edge conduction and bulk fine-tuning through the special transition in the quantum Hall effect. In the PRX study, Foster and co-authors Björn Sbierski of UC Berkeley and Jonas Karcher of KIT studied 3-D topological systems that are similar to the 2-D landscapes in the analogy.
"The interesting stuff in these 3-D systems is also only happening at the boundary," Foster said. "But now our boundaries aren't 1D edge states, they are 2-D surfaces."
Using "brute-force numerical calculations of the surface states," Sbierski, Karcher and Foster found a link between the critical 2-D quantum Hall state and the 3-D systems. Like the 1D edge state that persists above the transition energy in 2-D quantum Hall materials, the calculations revealed a persistent 2-D boundary state in the 3-D systems. And not just any 2-D state; it is exactly the same 2-D percolation state that gives rise to 1D quantum Hall edge states.
"What was a fine-tuned topological quantum phase transition in 2-D has been 'reincarnated' as the generic surface state for a higher dimensional bulk," Foster said. "In 2018 study, my group identified an analogous connection between a different, more exotic type of 2-D quantum Hall effect and the surface states of another class of 3-D topological superconductors. With this new evidence, we are now confident there is a deep topological reason for these connections, but at the moment the mathematics remain obscure."
Topological superconductors have yet to be realized experimentally, but physicists are trying to create them by adding impurities to topological insulators. This process, known as doping, has been widely used to make other types of unconventional superconductors from bulk insulators.
"We now have evidence that three of the five 3-D topological phases are tied to 2-D phases that are versions of the quantum Hall effect, and all three 3-D phases could be realized in 'topological superconductors,'" Foster said.
Foster said conventional wisdom in condensed matter physics has been that topological superconductors would each host only one protected 2-D surface state and all other states would be adversely affected by unavoidable imperfections in the solid-state materials used to make the superconductors.
But Sbierski, Karcher and Foster's calculations suggest that isn't the case.
"In quantum Hall, you can tune anywhere and still get this robust plateau in conductance, due to the 1D edge states," Foster said. "Our work suggests that is also the case in 3-D. We see stacks of critical states at different energy levels, and all of them are protected by this strange reincarnation of the 2-D quantum Hall transition state."
The authors also set the stage for experimental work to verify their findings, working out details of how the surface states of the 3-D phases should appear in various experimental probes.
"We provide precise statistical 'fingerprints' for the surface states of the topological phases," Foster said. "The actual wave functions are random, due to disorder, but their distributions are universal and match the quantum Hall transition."
More information: Björn Sbierski et al. Spectrum-Wide Quantum Criticality at the Surface of Class AIII Topological Phases: An "Energy Stack" of Integer Quantum Hall Plateau Transitions, Physical Review X (2020). DOI: 10.1103/PhysRevX.10.021025
Journal information: Physical Review X
Provided by Rice University