Weather applications of mathematical statistics

The application possibilities seem to be endless when it comes to mathematical statistics. Several of the researchers at the department work with issues related to weather, climate and environment – Igor Rychlik and David Bolin are two of them.

When you manufacture something, such as a car, a boat, an oil rig or an agricultural machine, you must take into consideration that it will be used in an environment that will affect its performance. Igor Rychlik develops models for such environments with the help of mathematical statistics, which are used by the manufacturers to simulate what their products will be exposed to.

The environment can for example involve various types of loads or weather conditions. Igor has worked extensively with models of significant wave heights, such as how big the waves are along the coast and out at sea, in the centre of a storm area and its outskirts, which directions they take and how they vary over long periods of time, such as ten years, seen from the perspective of an oil rig or a vessel. The forces that the ship or oil rig experience vary wave by wave and you need to know the variation of the forces, given the wave climate. The environment varies both in time and geography, and the ship will be used globally, hopefully during many years. At the same time simple models are sought, depending on as few parameters as possible, in order to easily be able to describe and classify the type of environment and the variation in the load environment that the ship might encounter. Igor's work is therefore a balance between simplicity and precision to get as relevant models as possible. Also, the research must be developed, so that the work not just will be consultancy assignments.

It is not just manufacturers who benefit from the wave models, also operators such as sea captains can use them to choose which course to take to reduce fuel consumption or avoid extreme loads. Igor collaborates with a number of organizations, such as Ifremer (L'Institut Français de Recherche pour l'Exploitation de la Mer) which has research activities on the sea around the world, Det Norske Veritas which classifies ships, and of course the Department of Shipping and Marine Technology here at Chalmers. Recently he has worked together with the US Coast Guard, where they with advanced mathematical methods calculated the risk of capsizing depending on the appearance of the wave as seen from the boat. It is a very special form of statistics, where also the speed of the boat affects.

Igor is now trying to develop a useful definition of length and width of waves. There are no generally accepted measures or consensus on how they should be indexed. If it is possible to find generally accepted ways to determine the size of for example storms and there are tools to calculate their distributions from mathematical models, it would also be possible to investigate whether they have changed over time, which of course is interesting for climate research in general. The main risk of the project is that the result is considered too complicated, and therefore is not used. Mathematics can be perceived as too difficult, while computer programs often are easier to understand and use, and can be a suitable way to get more people interested in the knowledge that has been developed. The many applications that have been developed over time in Igor's research group have been assembled in a toolbox, WAFO. There are statistical programs for wave and load descriptions, which are used pretty much out in the world.

Climate models and climate loads

David Bolin's research area is spatial statistics, i. e. analysis of data collected at different spatial locations. The application fields are numerous and diverse, at both micro and macro level, and David is involved in such seemingly different projects as modelling of the surface material in drug tablets as how to statistically correct climate models. The latter project is initiated by an insurance company, who wants to know if the rainfalls in a country will change. There exist global models for this but when refining them to regional models they fit poorly with the available data, and with the help from spatial statistics it is possible to correct these models so their output matches reality better.

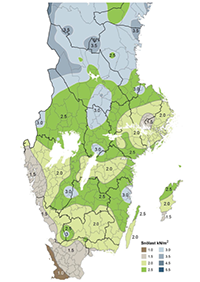
A similar problem that recently has been received from Boverket (National Board of Housing, Building and Planning), which is available as a suggestion for a master thesis, is about climate loads, namely the construction standards for how strongly roofs must be built to be able to withstand snow in different geographical areas. Boverket has provided such a map, and now wants to know whether this should be updated to give more realistic estimations of the anticipated snow loads (see pictures).

A project on a larger scale, which is more about climate than weather, is that related to the future sea level. Together with Peter Guttorp, who was a jubilee professor here one year ago, David produce projections of how the sea level will change as a result of global warming. There are many sources to that these projections are uncertain, such as how emissions will change in the future, and statistical methods are important here since these are used also to quantify the uncertainty in the projections.

David thinks that one fascination of the research area is the good possibilities to switch between interesting applications and method development. A methodological difficulty is the dependency between close measure points that must be taken into account and which makes the models more complicated than if data had been independent. Modelling is also often only one of the steps that must be handled in applications. A common problem in spatial statistics is to find areas that meet some certain criteria, as in the analysis of air pollutions where you often want to find areas that exceed some certain limit. To find areas of this kind is a difficult problem which is closely related to hypothesis testing. The difficulties lies partly in that it is not evident how the areas should be defined, partly in that they are very computationally demanding to estimate due to the spatial dependency in the models. So new ways must be found to tackle these problems, which also must be sufficiently computationally efficient so that they can be of practical use.