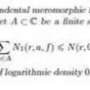A purported new mathematics proof is impenetrable – now what?

What happens when someone claims to have proved a famous conjecture? Well, it depends. When a paper is submitted, the journal editor will pass it off to a respected expert for examination. That referee will then scan the paper looking for a significant new idea. If there isn't one, then the whole argument is unlikely to get much more scrutiny.
But if there is a kernel of a new approach, it will be checked carefully. Additional experts may be consulted. Eventually the mathematics community may reach consensus that the argument is correct and the conjecture becomes a theorem. This can happen outside the formal refereeing process thanks to preprint servers such as the arXiv, but in the end, enough expert referees have to give the work their imprimatur before the paper is finally published in a journal.
In my mathematical career, there have been a few such big announcements, the most well-known being Andrew Wiles' solution of Fermat's Last Theorem in 1994. Grigori Perelman's proof in 2003 of the Poincaré Conjecture comes to mind as well. Now a reclusive yet respected Japanese mathematician has put forth a solution to another notorious problem.
In those earlier examples, the stature of the mathematicians involved made other experts interested in verifying their results. But what if the proposed solution is impenetrable? What if it reads, as University of Wisconsin Math Professor Jordan Ellenberg put it on his blog, like mathematics from the future, full of new concepts and definitions that are disconnected from current language and techniques? If the author is relatively unknown it may be dismissed, or even ignored. But if the mathematician has a reputation for being careful and producing solid results, what then?
The ABC conjecture
Shinichi Mochizuki of the Research Institute for Mathematical Sciences at Kyoto University is such a mathematician. In August 2012, he posted a series of four papers on his personal web page claiming to prove the ABC conjecture, an important outstanding problem in number theory. A proof would have Fermat's Last Theorem as a consequence (at least for large enough exponents), and given the difficulty of Wiles' proof of Fermat's Last Theorem, we should expect a proof of the ABC conjecture to be similarly opaque.
The conjecture is fairly easy to state. Suppose we have three positive integers a,b,c satisfying a+b=c and having no prime factors in common. Let d denote the product of the distinct prime factors of the product abc. Then the conjecture asserts roughly there are only finitely many such triples with c > d. Or, put another way, if a and b are built up from small prime factors then c is usually divisible only by large primes.
Here's a simple example. Take a=16, b=21, and c=37. In this case, d = 2x3x7x37 = 1554, which is greater than c. The ABC conjecture says that this happens almost all the time. There is plenty of numerical evidence to support the conjecture, and most experts in the field believe it to be true. But it hasn't been mathematically proven – yet.
Enter Mochizuki. His papers develop a subject he calls Inter-Universal Teichmüller Theory, and in this setting he proves a vast collection of results that culminate in a putative proof of the ABC conjecture. Full of definitions and new terminology invented by Mochizuki (there's something called a Frobenioid, for example), almost everyone who has attempted to read and understand it has given up in despair. Add to that Mochizuki's odd refusal to speak to the press or to travel to discuss his work and you would think the mathematical community would have given up on the papers by now, dismissing them as unlikely to be correct. And yet, his previous work is so careful and clever that the experts aren't quite ready to give up.
A meeting at Oxford
The Clay Mathematics Institute and the Mathematical Institute at Oxford recently sponsored a meeting about Mochizuki's work. He was not in attendance, but many of the world's leading number theorists and arithmetic geometers were. The goal was not to verify the proof of the ABC conjecture, but rather to equip experts in the field with enough background and information to at least begin to read through the papers carefully. There are many summaries of the meeting online (Stanford Math Professor Brian Conrad's is particularly detailed and illuminating), and some attendees tweeted about it.
The general feeling was one of frustration, especially during the last two days when audience members repeatedly asked for illustrative examples, were promised they were coming, but then they never materialized. Mathematicians have little patience for being led down a rabbit hole, but the potential payoff in this case may persuade some to at least go in a little deeper.
Prognosis
It's not clear what the future holds for Mochizuki's proof. A small handful of mathematicians claim to have read, understood and verified the argument; a much larger group remains completely baffled. The December workshop reinforced the community's desperate need for a translator, someone who can explain Mochizuki's strange new universe of ideas and provide concrete examples to illustrate the concepts. Until that happens, the status of the ABC conjecture will remain unclear.
There's a general sense among nonmathematicians that the subject is either right or wrong, and the truth is easily discovered. While our discipline does insist on rigorous, logical proof of correctness, we often argue over the details. This is good for mathematics since it generally leads to better exposition and streamlined proofs.
These arguments have happened before. Wiles' proof of Fermat's Last Theorem was scrutinized thoroughly, and an error was found which had to be corrected. Perelman's work on the Poincaré Conjecture was only a detailed sketch of a proof which required hard work on the part of others to be made rigorous. Mochizuki's work may eventually pass the test, but it could take many years before we get to a clean version that can be more widely understood.
Source: The Conversation
This story is published courtesy of The Conversation (under Creative Commons-Attribution/No derivatives).![]()