January 31, 2013 report
Quantum strategies fail to improve capacity of quantum optical communication channels
(Phys.org)—Quantum techniques have been demonstrated to offer improvements in areas such as computing, cryptography, and information processing, among others. But in a new study, researchers from IBM have proven that no quantum trick – no matter how complex or exotic – can improve the capacity of a type of quantum channel that serves as a building block of quantum optical communication systems. Although the result is somewhat surprising and a bit disappointing, it will help guide scientists to explore other ways to enhance channel capacity.
The researchers, Robert König and Graeme Smith at the IBM TJ Watson Research Center in Yorktown Heights, New York, have published a paper on the limits of quantum techniques for improving channel capacity in a recent issue of Nature Photonics.
As the researchers explained, channel capacity is operationally defined as the maximum achievable communication rate, measured in bits per channel use, and it provides an ideal way to gauge performance. The maximum capacity of classical communication channels can be determined by a simple formula derived by the famous mathematician and engineer Claude Shannon in the 1940s. But Shannon's formula does not account for quantum effects, such as entanglement. In the intervening decades, many researchers have thought that quantum mechanics could provide ways to increase the capacity of bosonic Gaussian channels, which would improve their speed and overall performance.
"Almost any communication link using electromagnetic signals is well described as a Gaussian channel for a wide range of parameters," Smith explained to Phys.org. "A Gaussian channel is basically a simple model for describing how a propagating electromagnetic field is corrupted as it propagates. A good example of this is communication in optical fiber.
"Researchers thought that quantum effects could improve the capacity of Gaussian channels because there are examples of more complicated channels (though somewhat contrived) where entangled signal states can be used to boost the capacity of the channel. Also, in terms of actually proving limits on the possible size of such an effect, there were huge gaps between the best known achievable rates and the best upper limits on the capacity. That gap looked like an opportunity."
However, efforts to improve channel capacity with quantum effects have fallen short. In the new study, König and Smith have finally shown why by providing the first mathematical proof showing that quantum strategies are essentially useless for increasing channel capacity; although the proof doesn't rule out some very small capacity increases, they would be too small to care about.
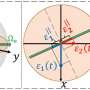
To reach this conclusion, the researchers considered the concept of entropy, which is a measure of a channel's noisiness and closely related to capacity. They mathematically showed that, when two quantum signals combine at a beamsplitter, then no matter what state each signal contains, the beamsplitter always increases entropy. Through their calculations, the researchers could determine an upper bound on the channel capacity that no quantum effect can improve upon. The results suggest that current technologies for increasing capacity in bosonic Gaussian channels are working at near optimal efficiency.
"Our results tell us, for all practical purposes, what the actual capacity of electromagnetic channels is and how we can come close to achieving it," Smith said. "The classical Gaussian noise channel was considered explicitly in Shannon's original work precisely because of its relevance for practical communication systems. Since many practical channels (such as optical) are well-described by the quantum Gaussian noise model, we know the best ways to go about using these resources. I should say also that the mathematical technique we developed, the quantum entropy power inequality, has a classical counterpart that has many applications in information, statistics, and deep mathematics. Our quantum entropy power inequality may have similar further applications to information theory and mathematics."
In order to keep pace with the explosion of data and delivery of bandwidth, researchers will have to turn to other strategies for improving communication technologies, such as using multi-mode fiber, multi-core fiber, or multi-level modulation, rather than quantum effects. König and Smith also plan to further investigate how the mathematical technique can be applied to other areas.
"I want to find more applications of the quantum entropy power inequality to the theory of quantum communications, perhaps in the network setting," Smith said. "It would also be great to find some appropriate mathematical generalizations and extensions and see where they lead. Like I said above, the classical version of the inequality has lots of unexpected connections to different parts of mathematics. I think the upper bound of the capacity can save people a lot of time by preventing them from looking unsuccessfully for quantum transmitters for this important channel."
More information:
Robert König and Graeme Smith. "Limits on classical communication from quantum entropy power inequalities." Nature Photonics. DOI: 10.1038/NPHOTON.2012.342
Also at arXiv:1205.3407 [quant-ph]
Journal information: Nature Photonics
© 2013 Phys.org