Semiconductor Lasers Generate Better Random Numbers

(PhysOrg.com) -- Random numbers -- numbers without any pattern -- are vital to many applications, such as computer simulations, statistics, and cryptography. There are many ways to generate them using unpredictable physical processes, including electric-signal noise and radioactive decay, but these methods cannot produce the quantities of numbers needed to keep up with the high data-processing rates of today's computers.

A group of scientists seems to have discovered a way around this problem. They have found that the physical chaos present in semiconductor lasers -- laser light produced using a semiconductor as the medium -- can produce good-quality random number sequences at very high rates.

The scientists, from Takushoku University, Saitama University, and NTT Corporation, all in Japan, achieved random number rates of up to 1.7 gigabits per second (Gbps), which is about 10 times higher than the second-best rate, produced using a physical phenomenon. They report this result in the December issue of Nature Photonics.

"We have shown that the performance of random number generators can be greatly improved by using chaotic laser devices," said the paper's corresponding author Atsushi Uchida, a researcher at Saitama University, to PhysOrg.com. "The rate we obtained is faster than that of any previously reported devices for generating random numbers using physical sources."

Fields and applications that could benefit from their work are numerous, including computational models to solve problems in nuclear medicine, computer graphic design, and finance. Random numbers are also important to internet security.

Generating random numbers using physical sources -- which can be as simple as coin-flipping and tossing dice -- are preferred over other methods, such as computer generation, because they yield nearly ideal random numbers: those that are unpredictable, unreproducible, and statistically unbiased.

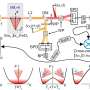
Lasers, Uchida and his colleagues have shown, can be excellent physical sources if they are chaotic. This is achieved, in this case, by reflecting part of the laser light back into the laser using an external reflector. This induces chaos, causing the light intensity to oscillate wildly. As a result, the light's electromagnetic signals are highly complex and cover a wide frequency range.

The researchers used a pair of semiconductor lasers in their experimental setup. Each laser is connected to a photodetector, a device that senses and measures light, and each photodetector is connected to an analog-to-digital converter (ADC), which samples the physical light signals and outputs digital numbers. In this case, the specific ADCs convert the signals into random binary numbers suitable for computing and other high-speed data manipulation.

The group achieved a bit rate of 1.7 Gbps, although future work may center on devising laser schemes that can achieving rates as high as 10 Gbps.

Citation: Atsushi Uchida, Kazuya Amano, Masaki Inoue, Kunihito Hirano, Sunao Naito, Hiroyuki Someya, Isao Oowada, Takayuki Kurashige, Masaru Shiki, Shigeru Yoshimori, Kazuyuki Yoshimura and Peter Davis Nature Photonics vol, 2, no. 12, pp. 728-732 (2008); DOI:10.1038/nphoton.2008.227

Copyright 2007 PhysOrg.com.
All rights reserved. This material may not be published, broadcast, rewritten or redistributed in whole or part without the express written permission of PhysOrg.com.

---

---