In quantum channels, zero plus zero can equal non-zero

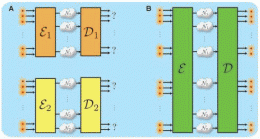
(PhysOrg.com) -- Physicists have discovered a strange characteristic of quantum communication channels. If two quantum channels each have a transmission capacity of zero, they may still have a nonzero capacity when used together. This effect, which has no classical counterpart, reveals a new complexity in the fundamental nature of quantum communication.

The coauthors of the study, Graeme Smith of the IBM T.J. Watson Research Center in Yorktown Heights, New York, and Jon Yard of Los Alamos National Laboratory in Los Alamos, New Mexico, have published their research in a recent issue of Science.

Smith and Yard explain that one of the most important challenges in designing communication networks of any kind is taking steps to correct for noise. By decreasing noise levels in communication channels, developers can increase channel capacity, which is defined as the number of bits (or qubits, in quantum channels) that one channel can transmit. For a channel with zero capacity, no bits are transmitted.

For several decades, scientists have used a well-known formula developed by Claude Shannon in 1948 for developing error-correction techniques in classical communication channels. This formula guides the design of modern communication schemes used in cell phones, the Internet, and deep-space communication. In this classical formula, capacity is additive: when two channels are used simultaneously to transmit data, the capacities of the channels are added to obtain the total capacity.

But even today, physicists don’t understand quantum communication nearly as well as the classical kind. In the current study, Smith and Yard show that some pairs of zero-capacity channels can have a positive quantum capacity when used together. As the physicists explain, that would be like two cut telephone cables being able to transmit data when used together. Their finding shows two things: that quantum capacity is not additive like classical capacity, and that the quantum capacity of a single channel does not completely specify its capability for transmitting quantum information.

“To me, the strange thing is that you have these two things that you would have thought were useless – I mean, you'd usually think that a zero-capacity channel was good for nothing – and when you put them together, somehow there's a kind of synergy and they develop a very quantifiable value,” Smith told PhysOrg.com. “This doesn't happen when you work with classical channels, and since my intuition was based on that case, I was really surprised when it happened here.”

The scientists account for this “superactivation” property by explaining that quantum channels have two different kinds of capacity. “Private capacity” is the rate at which a channel can send secure classical data. “Assisted capacity” is the transmission rate in which multiple symmetric channels can assist a given channel in sending quantum data.

As the physicists demonstrated, a channel’s assisted capacity is always at least half as large as its private capacity. As an example of superactivation, the scientists showed that combining a private “Horodecki” channel and a symmetric channel (each with zero capacity) can give a quantum capacity of more than 0.01 qubits per channel. It’s as if each channel has the potential to activate the other, canceling the other’s reason for having zero capacity.

“The effect has something to do with the existence of something called ‘private Horodecki channels,’” Smith said. “These channels have the weird property that, even though they're too noisy to allow quantum communication, somehow they still allow you to send classical messages that are completely private. Roughly speaking, we figured out that there's this second kind of channel – a symmetric channel – that also can't send any quantum messages on its own, but can be used to transform the private classical communication of the Horodecki channel into noiseless quantum communication.”

Superactivation raises some interesting questions about the nature of communication. For instance, you would think that the question “can this communication link transmit any information?” would have a straightforward answer. However, with quantum data, the answer may be that it depends on the context. If identifying a quantum channel’s capacity is not as straightforward as previously thought, then new ideas will be needed for designing error-correction techniques in quantum channels.

These results lead to other questions, such as what the effect of combing three channels might be. The overall complexity of quantum channel capacity will keep the researchers investigating the fundamental characteristics of communication in the physical world.

“First, I'd like to understand the role of privacy in this whole thing, and whether it's necessary to achieve a superactivation,” Smith said. “In the longer term, I'd like to see if we can turn the improved understanding of quantum error correction that comes out of this into some practical ways to reduce noise in prototypes for quantum computers.”

More information: Smith, Graeme and Yard, Jon. “Quantum Communication with Zero-Capacity Channels.” Science, 26 September 2008, Vol. 321. 10.1126/science.1164382.

Copyright 2008 PhysOrg.com.
All rights reserved. This material may not be published, broadcast, rewritten or redistributed in whole or part without the express written permission of PhysOrg.com.