How to avoid a sucker bet – with a little help from maths

Sitting in a bar, you start chatting to a man who issues you a challenge. He hands you five red and two black cards. After shuffling, you lay them on the bar, face down. He bets you that you cannot turn over three red cards. And to help you, he explains the odds.
When you draw the first card, the odds are 5-2 (five red cards, two black cards) in favour of picking a red card. The second draw is 4-2 (or 2-1) and the third draw is 3-2. Each time you draw a card the odds appear to be in your favour, in that you have more chance of drawing a red card than a black card. So, do you accept the bet?
If you answered yes, perhaps it's time for you to go over your maths. It's a foolish bet. The odds given above are only for a perfect draw. The real odds of you being able to carry out this feat are actually 5-2 against you. That is, for every seven times you play, you'll lose five times.
Odds against you
This type of bet is often called a proposition bet, which is defined as a wager on something that seems like a good idea, but for which the odds are actually against you, often very much against you, perhaps even making it impossible for you to win.
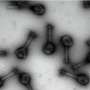
Let's assume that you took the bet and, almost inevitably, lost money. But this is just for fun, right? So your new "friend" suggests a way that you can get your money back. He takes two more red cards and hands them to you, so you now have seven red cards and two black cards. You shuffle the nine cards and lay them out, face down, in a three by three grid. He bets you even money that you can't pick out a straight line (vertical, horizontal or vertical) that has only red cards.
Intuitively, this might sound like a better bet and the odds are actually evens if the two black cards are next to each other in a corner (see image). In total there are eight lines to choose from and four contain only red cards, and four contain a black card. But that is as good as it gets.
If the black cards are in opposite corners then you can only win by choosing the centre horizontal or vertical row so the odds are 6-2 (or 3-1) against you winning. Every other layout gives you three winning lines and five losing lines. This bet only has 12 ways of succeeding, against 22 ways of you losing. Hardly an even-chance bet.
Have another go
Try to evaluate the odds for this proposition bet.
You shuffle a pack of cards and cut it into three piles. You are offered even money that one of the cards on top of the piles will be a picture card (a jack, queen or king). That is, if a picture card shows up, you lose. Do you think this is a good bet?
One way of reasoning is that there are only 12 losing cards against 40 winning cards, so the odds look better than evens? But this is the wrong way of looking at it. It is really what's known as a combinatorics problem. We should also realise that we are just choosing three cards at random.
There are 22,100 ways of choosing three cards from a 52 card deck. Of these, 12,220 will contain at least one picture card – so you lose – meaning that 9,880 will not contain a picture card – when you win. If you translate this to odds, you will lose fives times out of every nine times you play (5-4 against you). The even chance bet you have been offered is not the good value that you thought it was and you will lose money if you play a few times.
A Final Example
We can all agree that you have a 50/50 chance of guessing heads or tails in a coin toss. But if you toss the coin ten times, would you expect to see five heads and five tails? If you were offered odds of 2-1 to try this, would you take the bet? You'd be a sucker if you did.
Five heads and five tails will occur more often than any other combination, but there are many other ways that ten flips of a coin can land. In fact, the bet is 5-2 against you.
Another name for a proposition bet is the "sucker" bet, and there is no surprise who the sucker is. But don't feel too bad. We are all generally very poor at evaluating true odds. A famous example is the Monty Hall Problem. Even mathematicians could not agree on the right answer to this seemingly simple problem.
We have focused on bets where it is difficult, especially when under the pressure of deciding whether to bet or not, to calculate the true odds. But there are many other proposition bets that do not rely on calculating odds. And there are many other sucker bets, with probably the most famous being the Three Card Monty.
If faced with this type of bet, what is the best thing you can do? I'd suggest you simply walk away.
Provided by The Conversation
This article was originally published on The Conversation. Read the original article.![]()