Swimming upstream: Flux flow reverses for lattice bosons in a magnetic field

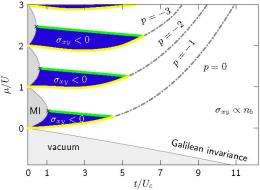
(PhysOrg.com) -- Matter in the subatomic realm is, well, a different matter. In the case of strongly correlated phases of matter, one of the most surprising findings has to do with a phenomenon known as the Hall response – an important theoretical and experimental tool for describing emergent charge carriers in strongly correlated systems, examples of which include high temperature superconductors and the quantum Hall effect. At Weizmann Institute of Science and California Institute of Technology, recent theoretical physics research into bosons interacting in a magnetic field has shown that, among other surprising effects, Hall conductivity – and therefore flux flow – undergo reversal. The scientists have concluded that their findings are immediately applicable to a wide range of phenomena in the realm of condensed matter physics.

Sebastian D. Huber in the Department of Condensed Matter Physics at Weizmann, working with Netanel H. Lindner at CalTech’s Institute for Quantum Information and Department of Physics, describes the obstacles encountered in conducting their research. “The Hall conductivity of continuum bosons is directly dictated by the density of particles,” says Huber. “Interesting lattice effects leading to a deviation from this elementary rule are only at work for strong inter-particle interactions. In short, the existence of holes is crucial to our work.”

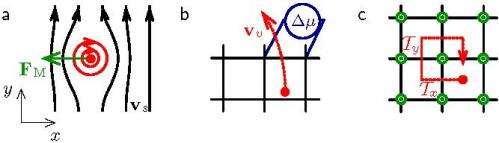
While for fermions the band theory of solids together with the Pauli principle provides the notion of holes, for bosons they needed an interaction-driven Mott insulator – a material that should conduct electricity according to conventional band theories, but due to particle-particle interactions is an insulator when measured, particularly at low temperatures – for holes to arise. “Hence, our main challenge was to study a lattice effect in the presence of strong interactions.”

Huber and Lindner addressed the question of the Hall conductivity by using concepts of topology like the Chern number and the effective magnetic monopoles which constitute sources of the Chern density. “While these concepts are well known,” Huber explains, “their application to gapless interacting systems is novel. The realization that gapless systems can also have topological transitions like the ones we found between different values of the Chern number, or equivalently, the Hall conductivity, is certainly an exciting new discovery. The actual question we resolved - the value of the Hall coefficient of lattice bosons – has been a longstanding problem motivated by high-temperature superconductivity. In this sense, our work contributes to a deeper understanding of lattice systems in general.”

Huber describes the next steps being considered, given that their work has shown that sign reversals of the Hall conductivity are possible in clean systems with no disorder, as well as in a purely bosonic model. “In high-temperature superconductors such reversals are experimentally observed,” Huber notes. ”The common wisdom is that the underlying Fermi surface is undergoing a structural change due to a competing instability of strip-formation. Our work suggests that this might not be the only source of such sign-reversals and no fermionic mechanism needs to be invoked. As the exact nature of these systems is highly controversial, we plan to extend our work to be able to directly access this problem.”

Concerning the surprising effects they found (e.g., sign reversal) of topological transitions between different integer values, Huber stresses that while it has been well-known since the discovery of the quantum Hall state that topology can have an important influence on solid state systems, there has recently been tremendous interest in topologically non-trivial states in the form of topological insulators. “However,” he adds, “all these systems are characterized by a gap to excitations in the bulk. Our results show that such topological transitions, and consequently the sign-reversals, are possible also in a gapless superfluid.”

While these findings are new, they are actually based on a very old theorem by von Neumann and Wigner regarding level crossings. “So,” Huber concludes, “in a sense we only brought existing knowledge on the structure of energy levels into the fascinating new world of topology in condensed matter systems.”

Copyright 2011 PhysOrg.com.
All rights reserved. This material may not be published, broadcast, rewritten or redistributed in whole or part without the express written permission of PhysOrg.com.