New tool can help predict the next financial bubble

An international team of interdisciplinary researchers has identified mathematical metrics to characterize the fragility of financial markets. Their paper "Network geometry and market instability" sheds light on the higher-order architecture of financial systems and allows analysts to identify systemic risks like market bubbles or crashes.

With the recent rush of small investors into so-called 'meme stocks' and reemerging interest in cryptocurrencies, talk of market instability, rising volatility, and bursting bubbles is surging. However, "traditional economic theories cannot foresee events like the US subprime mortgage collapse of 2007" according to study author Areejit Samal. He and his colleagues from more than ten mathematics, physics, economics, and complex systems focused institutions around the globe have made a great stride in characterizing stock market instability.

Their paper abstracts the complexity of the financial market into a network of stocks and employs geometry-inspired network measures to gauge market fragility and financial dynamics. They analyzed and contrasted the stock market networks for the USA S&P500 and the Japanese Nikkei-225 indices for a 32-year period (1985-2016) and for the first time were able to show that several discrete Ricci curvatures are excellent indicators of market instabilities. The work was recently published in the Royal Society Open Science journal and allows analysts to distinguish between 'business-as-usual' periods and times of fragility like bubbles or market crashes.

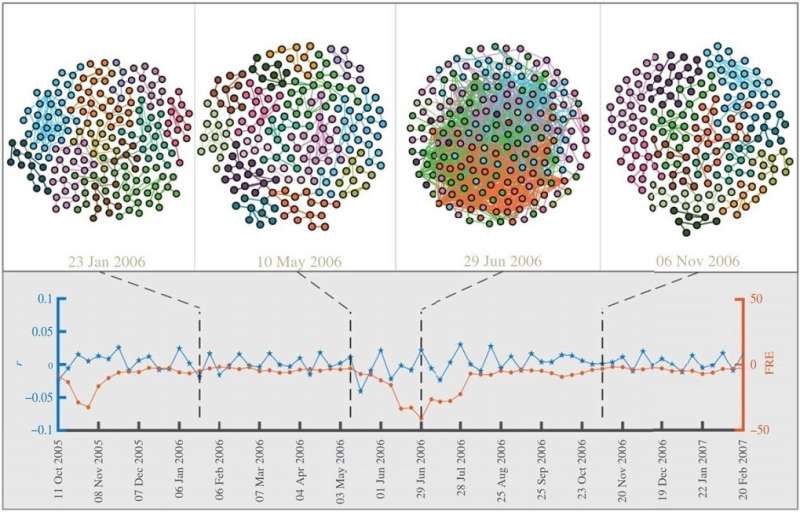
The network created by connecting stocks with highly correlated prices and trading volumes forms the structural basis of their work. The researchers then employ four discrete curvatures, developed by the director of Max Planck Institute for Mathematics in the Sciences Jürgen Jost and his coworkers, to study the changes in the structure of stock market networks over time. Their comparisons to other market stability metrics have shown that their four notions of curvature serve as generic indicators of market instability.

One curvature candidate, the Forman-Ricci curvature (FRE), has a particularly high correlation with traditional financial indicators and can accurately capture market fear (volatility) and fragility (risk). Their study confirms that in normal trading periods the market is very fragmented, whereas in times of bubbles and impending market crashes correlations between stocks become more uniform and highly interconnected. The FRE is sensitive to both sector-driven and global market fluctuations and whereas common indicators like the returns remain inconspicuous, network curvatures expose these dynamics and reach extreme values during a bubble. Thus, the FRE can capture the interdependencies within and between sectors that facilitate the spreading of perturbations and increase the danger of market crashes.

Max Planck Institute for Mathematics in the Sciences director Jürgen Jost summarizes the struggle of analyzing market fragility: "there are no easy definitions of a market crash or bubble and merely monitoring established market indices or log-returns does not suffice, but our methodology offers a powerful tool for continuously scanning market risk and thus the health of the financial system." The insights gained by this study can help decision-makers to better understand systemic risk and identify tipping points, which can potentially forecast coming financial crises or possibly even avoid them altogether.