August 17, 2020 feature
Ripple effects after slow-motion bubble collapse

A recent feature cover photo on Science portrayed a bubble in mid-collapse, based on a study conducted by Alexandros T. Oratis et al. The research team in mechanical engineering, mathematics and aerospace engineering at Boston University, MIT and Princeton University demonstrated the formation of intriguing wave-like patterns when bubbles underwent collapse. Using a complex lighting setup and fast shutter speed in the lab, perfectly aligned to capture a fleeting moment, within one second, they photographed the tiny bubble emerging from the surrounding media of dense silicone oil.
The rupture and collapse of viscous bubbles are widespread in nature and in industrial applications. The phenomenon is accompanied by elastic sheets that develop radial wrinkles. While the weight of the film appeared to play a dominant role during film collapse and wrinkling instability, in this work, gravity appeared to play a surprisingly negligible role. Based on fluid mechanics of the phenomena, Oratis et al. showed surface tension to be the driving factor during collapse to initiate dynamic buckling instability and wrinkling behavior, accompanied with the breakdown of curved viscous and viscoelastic films. The research work is relevant to understand industrial and chemical applications, including aerosol production from exhalation events in the respiratory tract.
Wrinkling of thin sheets
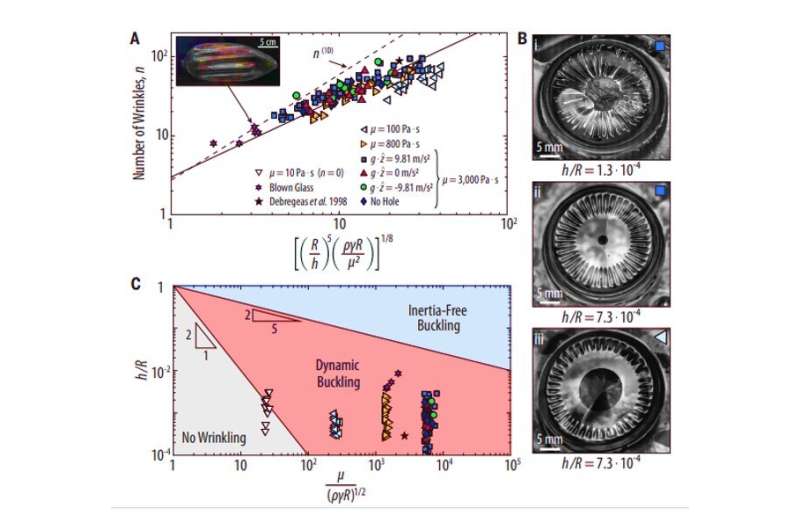
Understanding the formation of bubbles is important due to their ubiquity in nature and industrial applications including bubble collection during glass manufacture, spray painting, disposal of radioactive waste and in volcanic eruptions. Elastic sheets can wrinkle under compressive stress since they require less energy to buckle than compress. In recent studies researchers have focused on understanding the bending deformations that occur when a thin elastic sheet is stretched, poked, or wrapped around a curved object. Similarly, viscous liquids too can buckle in a process observed as 'parachute instability' when a rising bubble reaches the surface to rupture. After surfacing, a bubble consists of a thin liquid film in the form of a spherical cap supported by the gas trapped inside it. The wrinkles that develop during bubble rupture do so due to the weight of the collapsing thin film to allow trapped gas to escape. Oratis et al. showed that the wrinkling instability did not specifically depend on gravity or the presence of a hole experimentally formed to allow trapped gas to escape from the bubble.

Proof-of-concept
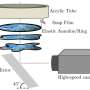
The team conducted experiments and observed the development of wrinkles in a collapsing bubble on a silicon oil bath to show how it was driven by surface tension instead of gravity. To test the hypothesis, they conducted an experiment with bubbles turned upside down, an approach facilitated due to the liquid viscosity. They accomplished this by preparing the bubble right-side up and rapidly rotating the sample to rupture it in a few seconds. When inverted, the bubble film continued to maintain its shape and thickness at the apex. If gravity and viscosity had been dominant contributors to the process, the inverted bubbles would have elongated downward as seen in simulations. Instead, the team noted the inverted bubble reverting back up against the force of gravity, while wrinkles formed during the final stages of bubble collapse, providing them a clear view of the process.
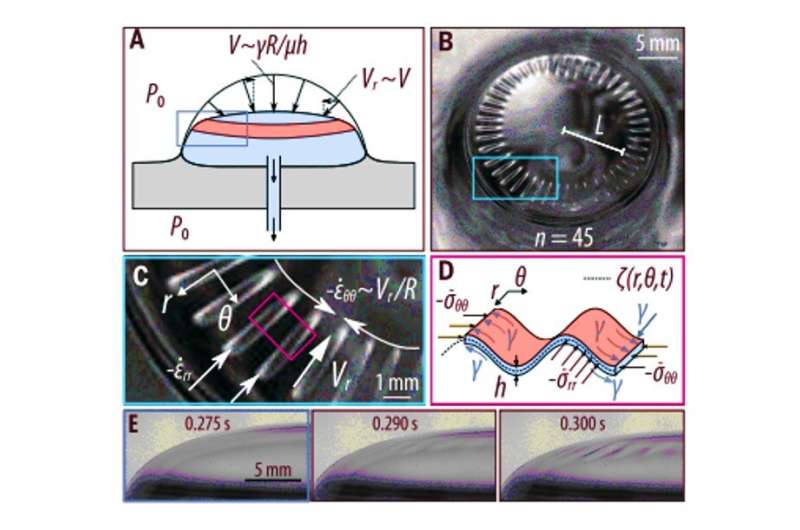
Surface tension—the driving force
To understand surface tension, the driving force behind the phenomenon, the scientists measured key parameters characteristic of the time-scale of collapse. For this, Oratis et al. used silicon oils with various viscosities and varied film thicknesses during the experiments. Using high-speed images, they calculated representative velocity at the onset of wrinkling and increased the viscosity of the silicone oil, to slow down collapse. As expected, thinner bubbles collapsed faster. The model derived in this work showed how the number of wrinkles strongly depended on the size of the hole created to initiate bubble collapse. During experimental demonstrations, the team eliminated the pressure difference across the bubble surface using a capillary-driven setup that did not breakdown the thin film, as a result, the hole created in the process efficiently induced bubble collapse without rupturing the film.

The experimental results were in fair agreement with the theory. The competition between tensile stress and compressive stress in the system affected the location of wrinkling patterns in the sheets. Oratis et al. performed additional experiments with thicker structures using blown molten glass extracted from the furnace, where they allowed the trapped air to escape through the glass-blowing pipe. During the process, the blown glass collapsed to adopt the shape of a wrinkle. The model derived in this work had limitations for data with the thinnest films where the collapse was so abrupt that the wrinkling pattern lost its symmetry to span the entirety of the bubble. Furthermore, the model predicted that wrinkling would not occur for all conditions.
In this way, Oratis and colleagues showed that surface tension, not gravity, drove the collapse of viscous surface bubbles. They developed a capillary-driven collapse system to initiate dynamic buckling instability via the simultaneous interplay of inertia, compression, and viscous binding of the relapsing film. The work presented viscous sheets with elastic-like instabilities during rapid compression. The results can also explain the fluid mechanics of the exhalation of potential pathogen-bearing aerosols that are linked to the breakdown of thin bubble films in the viscoelastic fluid that lines the respiratory tract. The present work suggests that surface tension alone may prompt buckling instability during viscous film rupture for these films to fold and entrap air, thereby providing deeper insight to the mechanisms of aerosolization.
More information: Oratis A. et al. A new wrinkle on liquid sheets: Turning the mechanism of viscous bubble collapse upside down, Science, 10.1126/science.aba0593
F. E. C. Culick. Comments on a Ruptured Soap Film, Journal of Applied Physics (2005). DOI: 10.1063/1.1735765
B. Davidovitch et al. Prototypical model for tensional wrinkling in thin sheets, Proceedings of the National Academy of Sciences (2011). DOI: 10.1073/pnas.1108553108
Journal information: Science , Journal of Applied Physics , Proceedings of the National Academy of Sciences
© 2020 Science X Network