Weird mathematical method holds up to testing

Twenty-two years after it was first proposed, mathematicians from Massey University, New Zealand, the Norwegian University of Science and Technology, Norway and La Trobe University, Australia have demonstrated why an unconventional mathematical method –critical for computer programming – works so well.

Differential equations are powerful mathematical equations that show the relationship between physical quantities (functions) and their rates of change (derivatives). For example, they can be used to describe heat transfer in a pump, or predator-prey relationships over time.

The Kahan method (named after Dr William Kahan) describes a process for breaking up differential equations so they can be solved numerically – a process called discretising. The process is vital for building computer packages, as programmes need to be able to run through a problem step by step.

The method was originally developed to be used only with quadratic equations. But in a paper published today in the prestigious Proceedings of the Royal Society A - Mathematical and Physical Sciences, authors demonstrate that it can be used for a range of equations.

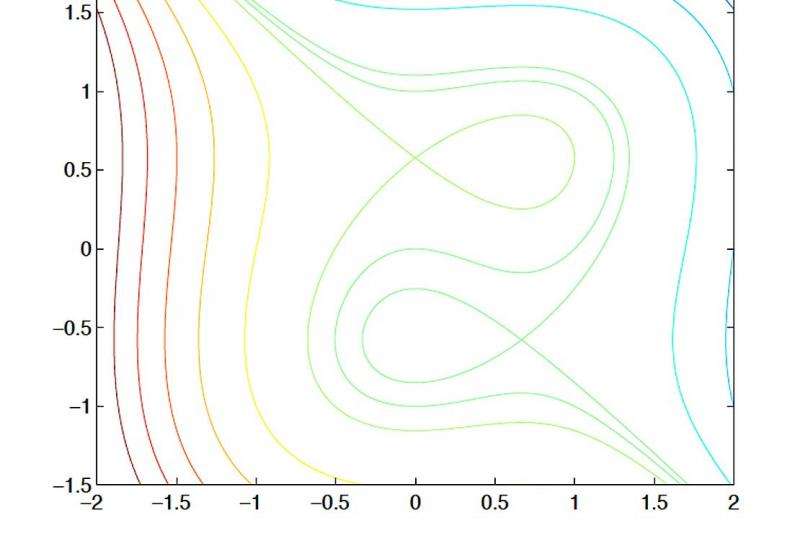
Professor of Applied Mathematics Robert McLachlan used an approach known as geometric integration, which preserves some of the geometric features of the original equation to more accurately predict outcomes, particularly long term.

He says the Kahan method has been accepted as being remarkably good for discretising a number of equations, but it was not known why – even by the inventor.

"Kahan noted he had used the method for 24 years without quite understanding why it works so well as it does. You can only understand it by putting it in a wider context. This is a situation we're faced with all the time in mathematics."