Scientists observe quantum superconductor-metal transition and superconducting glass
An article published in Nature Physics on March 30, 2014, presents the results of the first experimental study of graphene-based quantum phase transition of the "superconductor-to-metal" type, i.e. transformation of the system's ground state from superconducting to metallic, upon changing the electron concentration in graphene sheet.
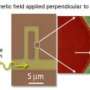
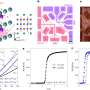
The system is a regular array of tin nanodisks (the radius of each disk is 200 nm) situated on a graphene substrate. Tin becomes a superconductor at temperatures lower than T0 = 3.5 degrees Kelvin. Tin nanodiscs electrically contact with each other due to electronic conductivity through graphene. At temperatures significantly below T0 the state of the nanodisk can be characterized by a single variable - "phase," defined in the period from 0 to 2π. Due to the transfer of Cooper pairs of electrons between nanodiscs, the so-called Josephson junctions are formed, which seek to establish a coherent superconducting state with uniform nanodisk phases across the entire lattice.
Graphene gradually changes the density of conduction electrons by changing the voltage on the electrostatic gate, and thus the strength of Josephson junctions between tin nanodiscs. Phase correlations among nanodiscs are destroyed by thermal fluctuations at temperatures above the critical temperature Tc. At high density of conduction electrons in graphene, the measured value Tc (around 0.5-0.7 K) is in good agreement with the previously developed theory, published in the 2009 article "Theory of proximity-induced superconductivity in graphene," in Solid State Communications.
Upon lowering the electron density of graphene, the energies of Josephson junctions weaken due to increase in the resistance of graphene, and the temperature of transition into coherent state drops sharply to below the minimum temperature of the experiment (60 mK). In other words, the spatial phase coherence between different individual nanodisks is destroyed solely by quantum (independent of temperature) phase fluctuations. As a result, superconductor-to-metal quantum phase transition takes place.
First approach to the theory of such a phase transition have previously been developed in the paper Feigel'man, M.; Larkin A. & Skvortsov, M. "Quantum superconductor-metal transition in a proximity array," Physical Review Letters *86* 1869, (2001).
In the domain of lowest measurable temperatures the resistance of the studied array turns out to be nearly temperature-independent, and, at the same time, it is an exponentially sharp function of voltage on the electric back-gate; this observation has yet to be explained, as no complete theory is capable of describing it at present.
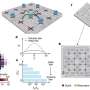
In addition to the above-mentioned superconductor-to-metal transition, the authors discovered the so-called "superconducting glass" state, which is created as a result of disorder and frustration in the Josephson junctions, but nevertheless corresponds to some of the minima of the total energy of the Josephson junctions array. Here, the controlling parameter is the strength of external magnetic field. Competition of periodic dependency on the magnitude of magnetic flux through the elementary cell of the nanodisk lattice and random dependency on the same parameter (due to mesoscopic fluctuations) leads to a phase diagram of the "re-entrant" type. Namely, the magnitude of the maximum superconducting current that flows through the entire lattice depends non-monotonically upon an external magnetic field; first it decreases (all the way down to zero), and then reappears with the increase of the magnetic field in a certain range of its values.
More information: "Collapse of superconductivity in a hybrid tin–grapheme Josephson junction array'," Zheng Han, Adrien Allain, Hadi Arjmandi-Tash,Konstantin Tikhonov, Mikhail Feigelman, Benjamin Sacépé,Vincent Bouchiat, published in Nature Physics on March 30, 2014, DOI: 10.1038/NPHYS2929
M.V. Feigel'man, M.A. Skvortsov, K.S. Tikhonov, "Theory of proximity-induced superconductivity in graphene," Solid State Communications, Volume 149, Issues 27–28, July 2009, Pages 1101-1105, ISSN 0038-1098, dx.doi.org/10.1016/j.ssc.2009.02.049.
Journal information: Nature Physics , Physical Review Letters
Provided by Moscow Institute of Physics and Technology